Q.6. Find the volume of the solid formed by revolving the region bounded by the graph of the equation y = x , y = 0 and
x = 4, about the x-axis.
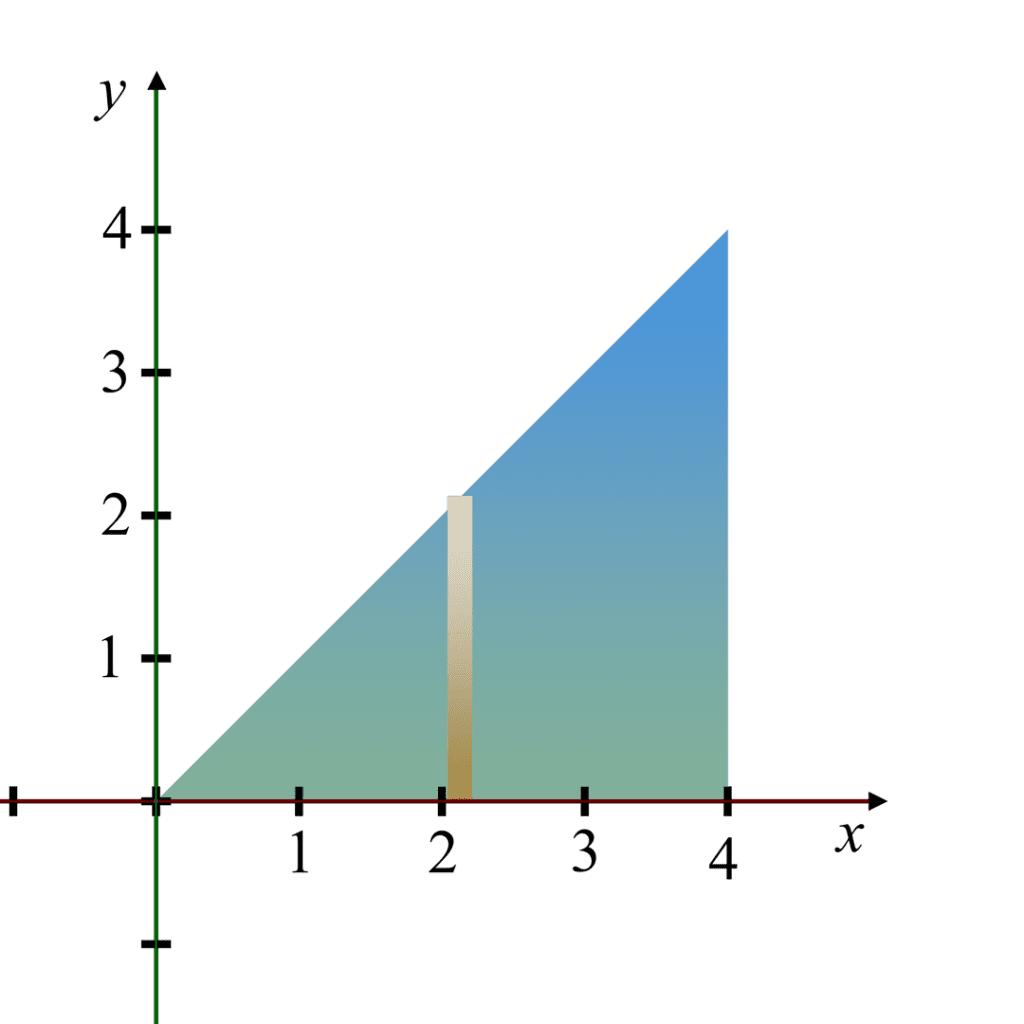
Recall that the volume of a solid formed when a curve is revolved about the x-axis is given by
Taking the constant (π) outside of the integral, we get:
Here, f(x) is the given function, which is y = x. And since we are finding the volume of the solid formed when this curve is revolved about the x-axis from x = 0 to x = 4, the integral becomes:
Integrating, we get:
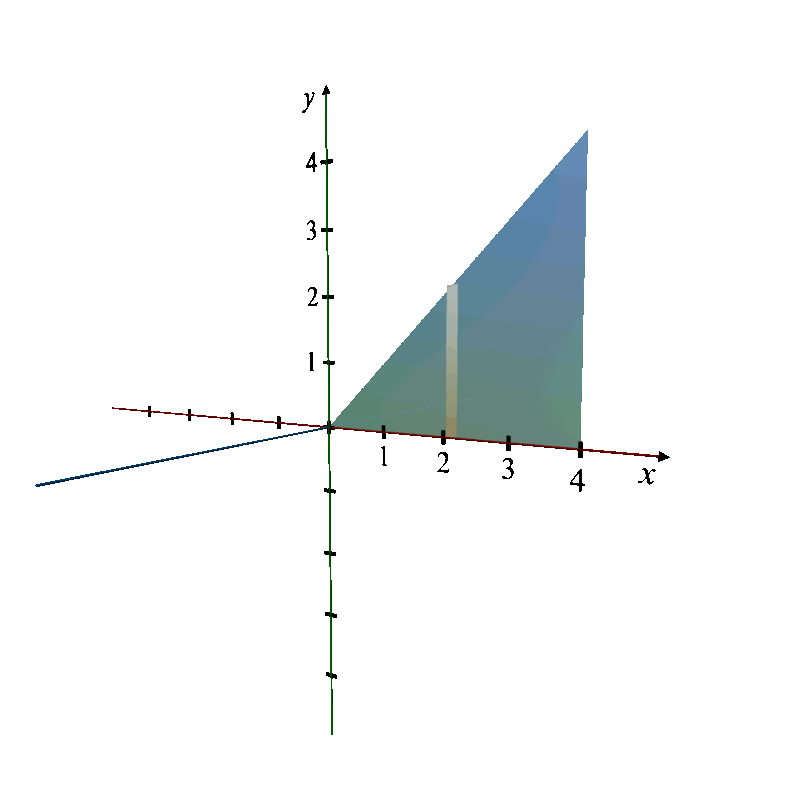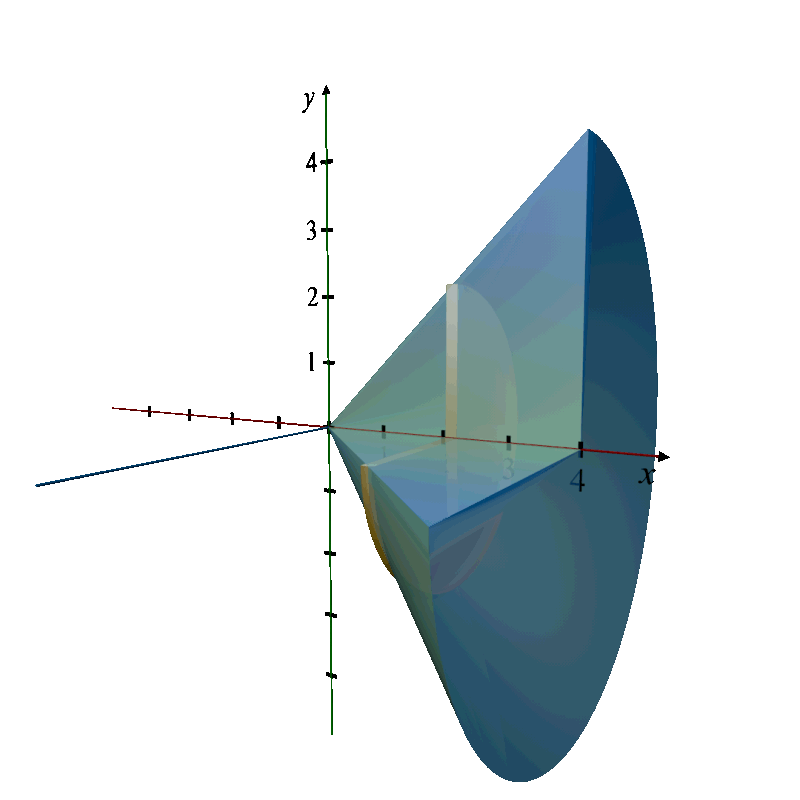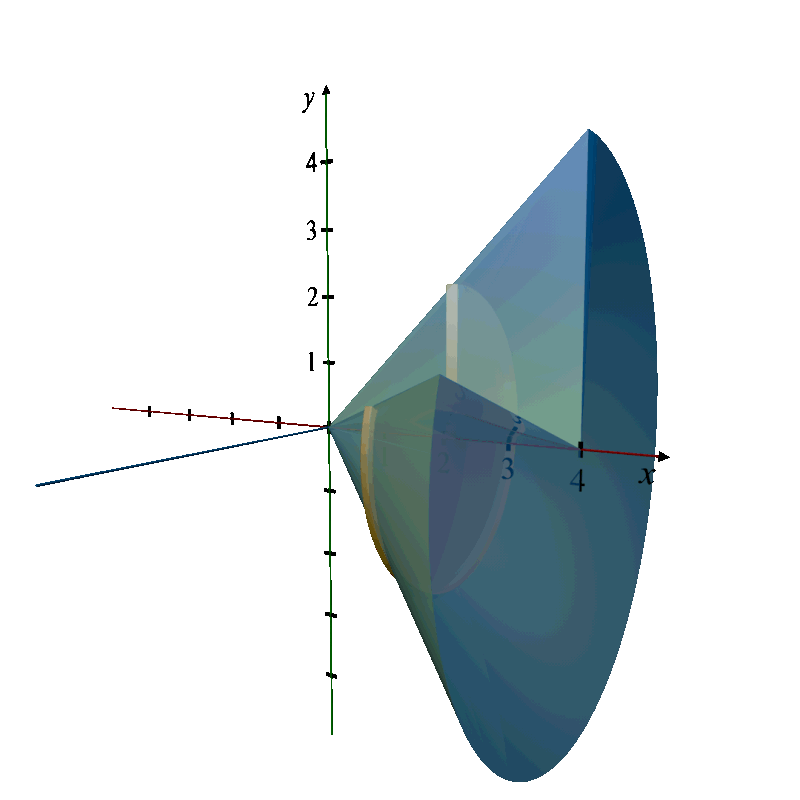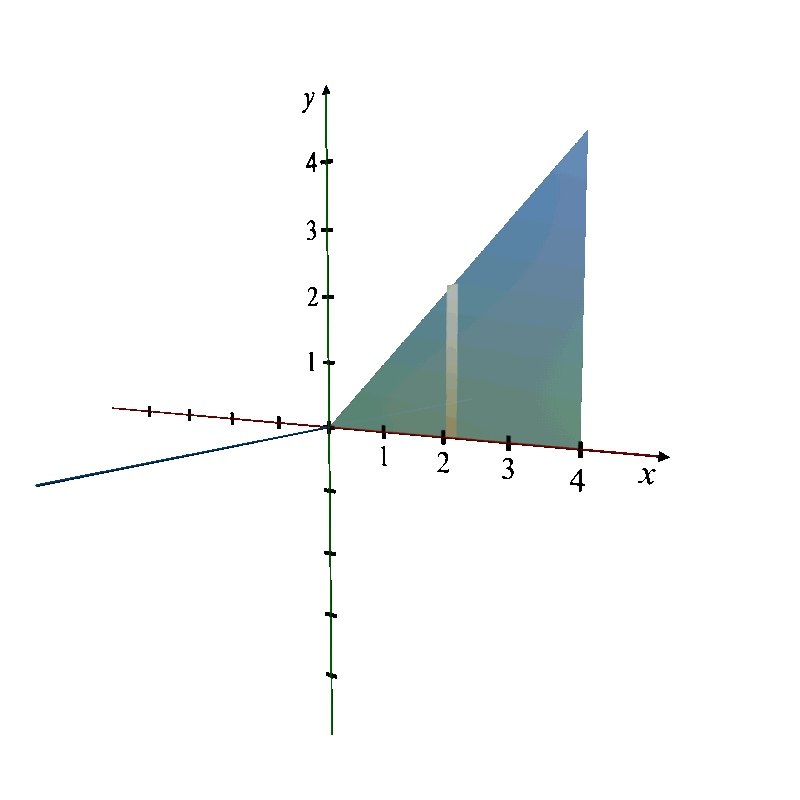
Shape Description:
The region described is a right triangle formed by the lines
𝑦 = 𝑥, 𝑦 = 0, and 𝑥 = 4. The line 𝑦 = 𝑥 represents the diagonal from the origin (0,0) to the point (4,4) on the graph.
The line 𝑦 = 0 is the x-axis, and 𝑥 = 4 is a vertical line cutting upward at
𝑥 = 4.
Volumes Of Revolution
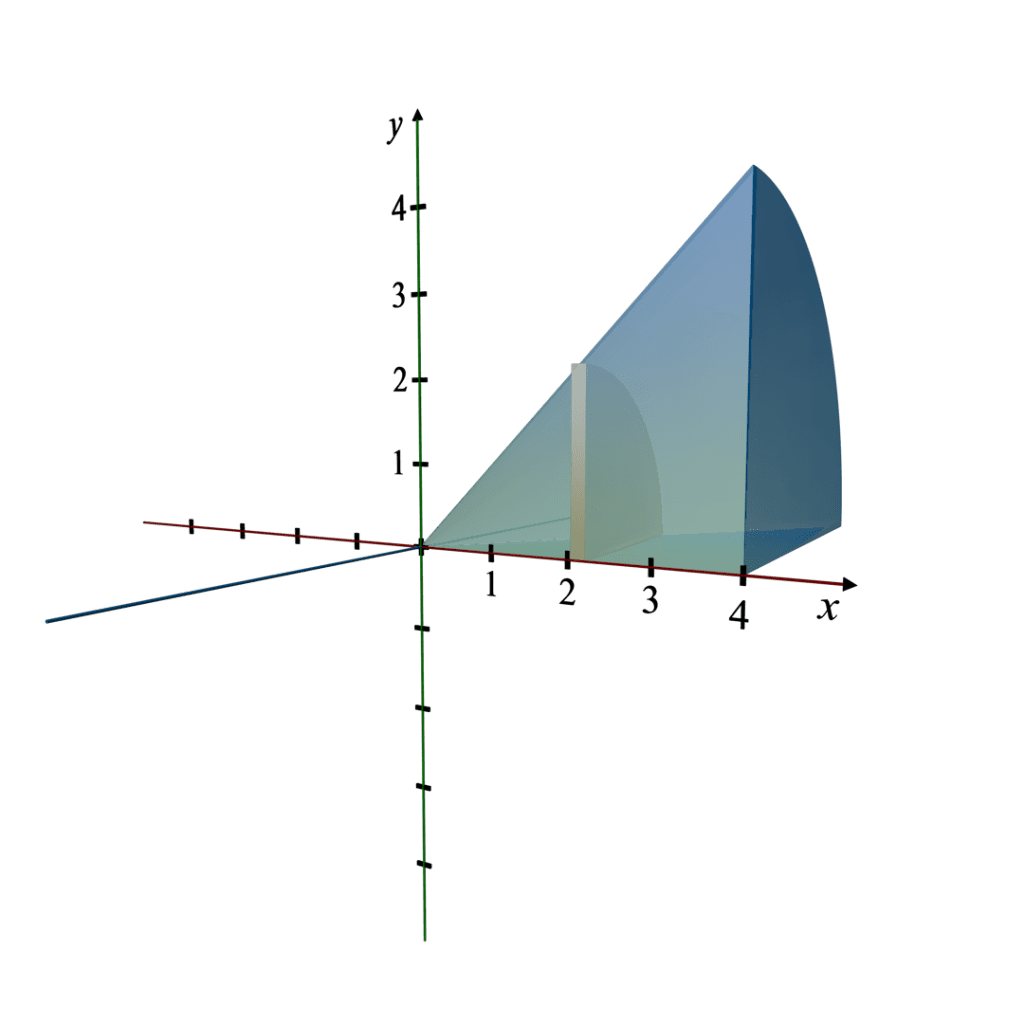
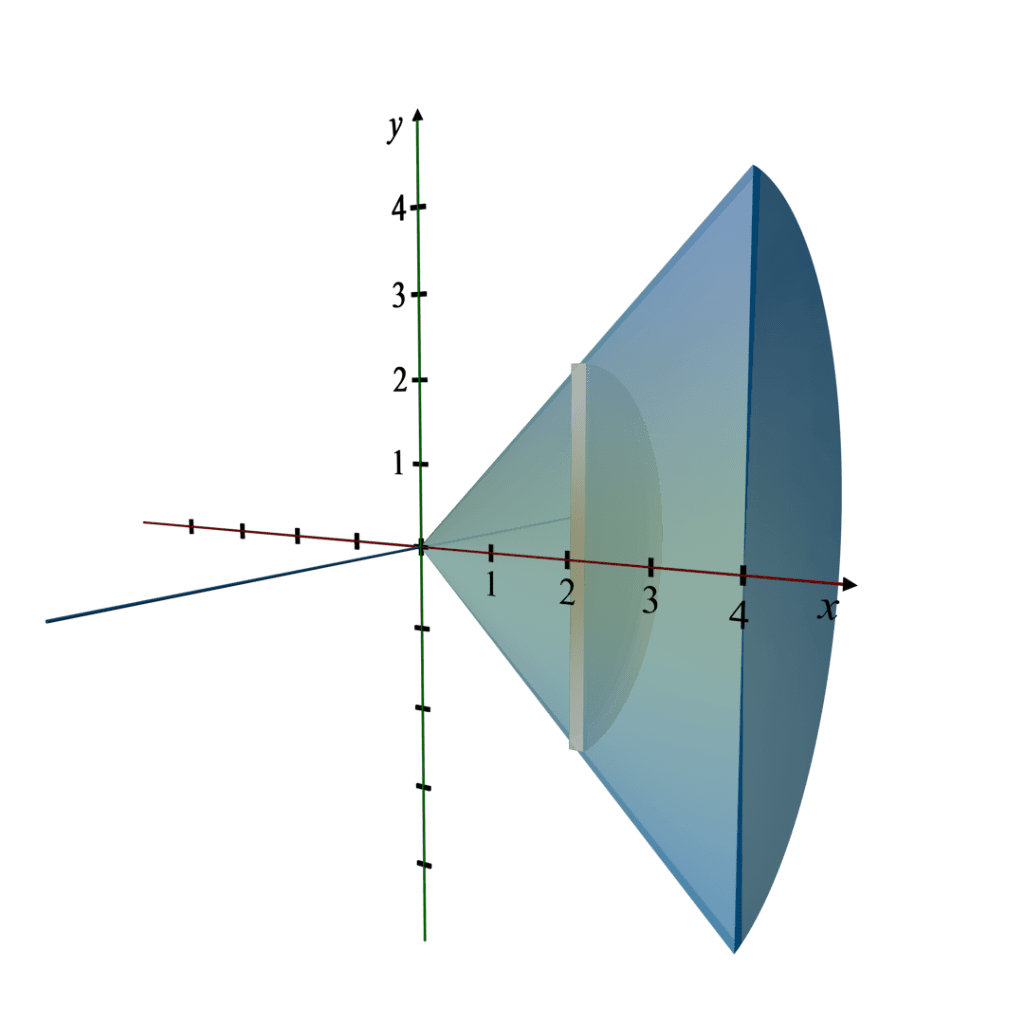
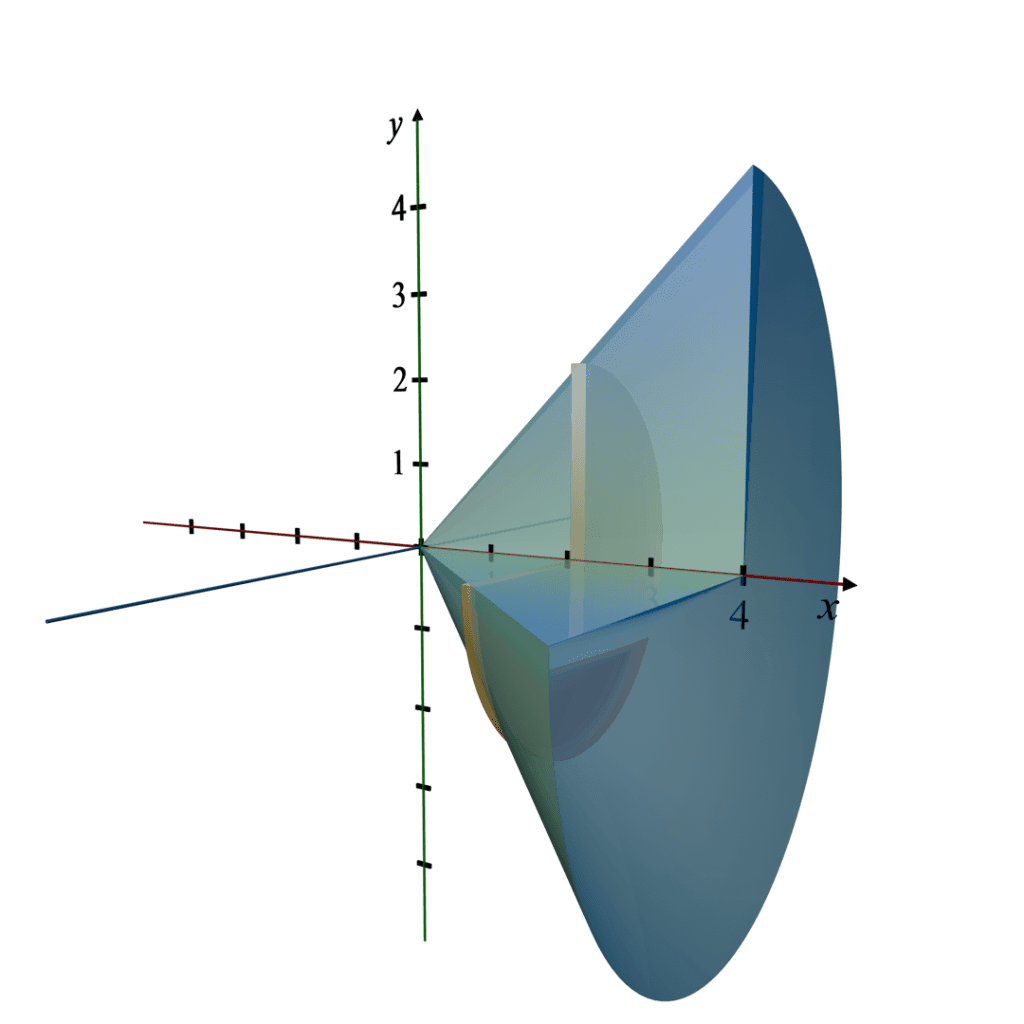
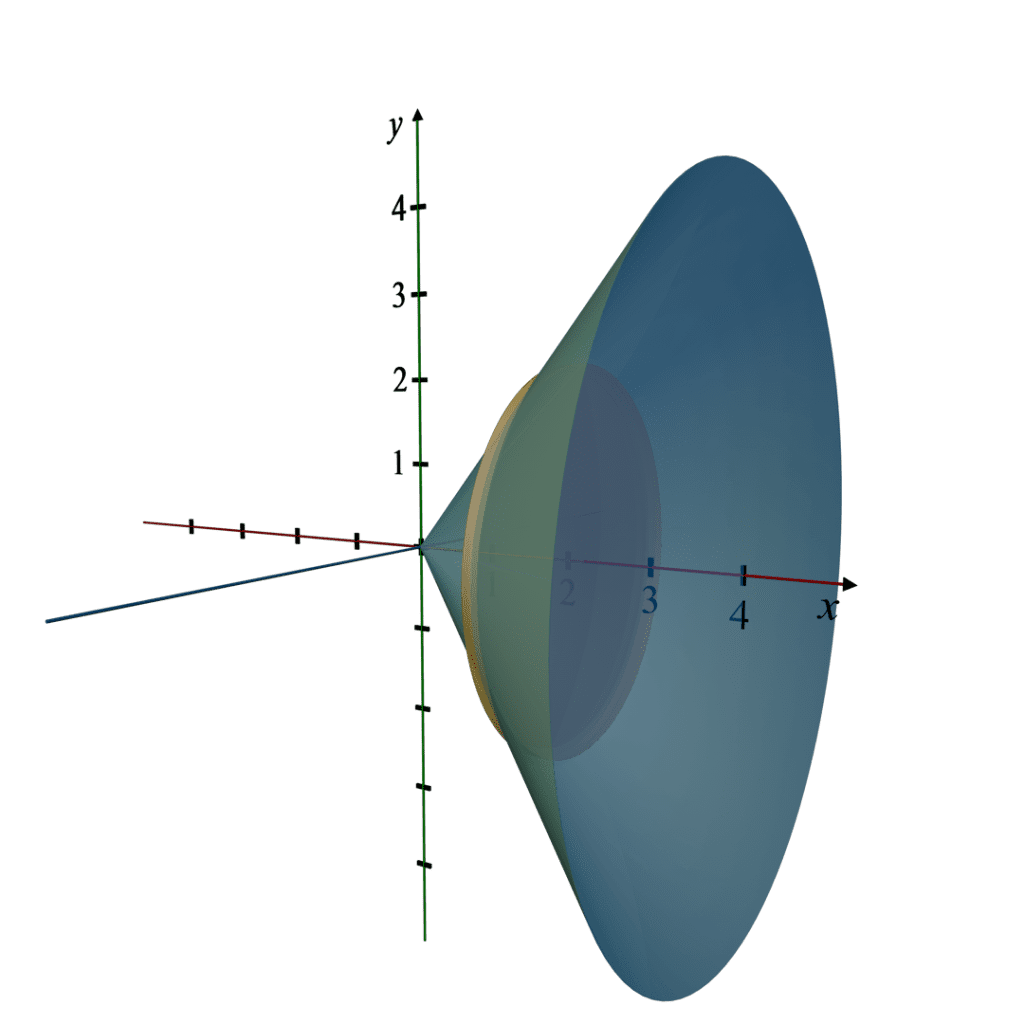
3D Revolution Description:
When this triangular region is revolved around the x-axis, it forms a solid known as a cone. The base of the cone lies on the x-axis, stretching from the origin to the point 𝑥 = 4, and it has a radius of 4 units (since the height at 𝑥 = 4 is 4). The apex of the cone is at the origin, and it extends along the x-axis up to 𝑥 = 4
Application of Volumes of Revolution
Volumes of revolution are applied in various fields such as engineering, physics, and medicine to solve practical problems involving the creation of 3D objects from 2D shapes. Here are some applications:
1. Engineering
Design and Manufacturing: Engineers often use volumes of revolution to design and manufacture parts with rotational symmetry, such as gears, wheels, and axles. For example, when designing a car wheel, the profile of the wheel can be rotated around the central axis to create the 3D shape of the wheel.
Aerospace Engineering: The fuselage of an aircraft can be modeled as a volume of revolution. By rotating the cross-sectional profile of the fuselage around the longitudinal axis, engineers can calculate the volume and surface area, which are critical for determining material requirements and aerodynamic properties.
2. Physics
Fluid Dynamics: In fluid dynamics, the shape of a container can affect how fluids behave within it. For example, the shape of a wine glass can be created by revolving a curve around an axis, and the volume of the wine glass can be calculated using the disk or washer method to determine how much liquid it can hold.
Solids of Revolution in Mechanics: Objects such as flywheels or pulleys, which have rotational symmetry, are often analyzed using volumes of revolution to determine their mass distribution and moments of inertia.
3. Medicine
Medical Imaging: Volumes of revolution are used in medical imaging techniques such as MRI and CT scans to create 3D models of organs from 2D cross-sectional images. This helps doctors visualize and analyze the structure of organs for diagnostic and surgical planning purposes.
Prosthetics and Implants: Designing prosthetic limbs and implants often involves creating objects with rotational symmetry. By using volumes of revolution, designers can calculate the required volume and shape to ensure proper fit and function.
Graphs of Inverse Trigonometry Functions
AREAS btw CURVES and VOLUMES of REVOLUTIONS
Word to JPEG – Convert Word files to JPEG Images Online
WebP to JPEG – Convert WebP to JPEG
HEIC to JPG – Convert HEIC Images to JPEG Format