3.4 Capacitors in a Circuit

3.4 Capacitors in a Circuit

Capacitors in Circuits: Comprehensive Guide

Capacitors are vital components in electrical circuits, frequently appearing in circuit analysis and applications. If you encounter a free-response question (FRQ) in exams, especially in AP Physics, there is a strong likelihood it will involve capacitors. Understanding their behavior and applications is key to mastering circuit analysis. This guide explores capacitors in series and parallel configurations, their behavior in steady-state circuits, and the functionality of RC circuits.

Capacitors in Series and Parallel

Parallel Capacitors

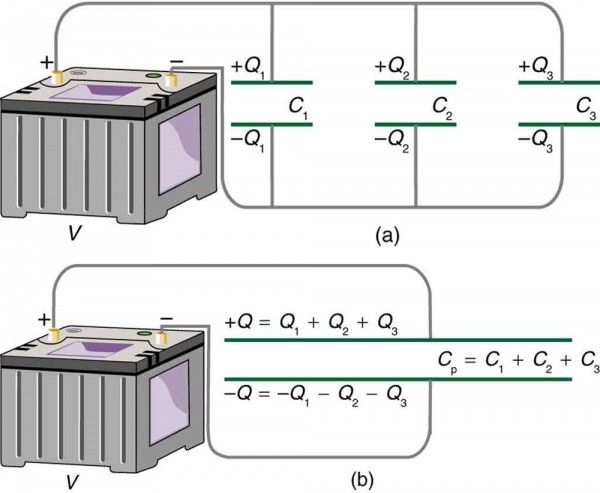
In parallel circuits, capacitors effectively behave as a single large capacitor. The total charge stored by the circuit is the sum of the charges on individual capacitors. The equivalent capacitance (ΣC) for capacitors in parallel is:

Parallel capacitors are ideal for storing large amounts of charge because their combined capacitance increases with every added capacitor.

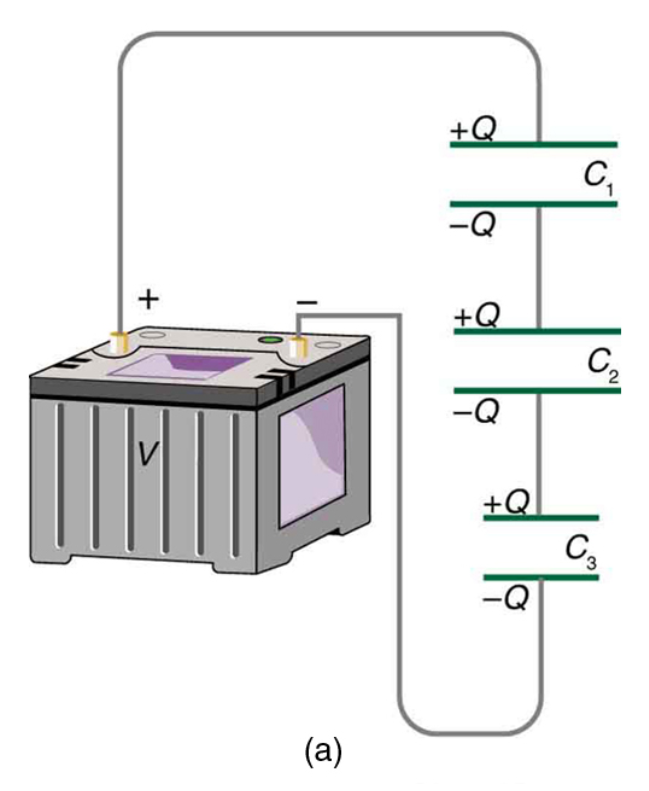
Series Capacitors

In a series configuration, capacitors share the same charge, but the total voltage across the series is the sum of the voltages across each capacitor. The equivalent capacitance (ΣC) is calculated using:

Series capacitors are used in applications where the voltage must be divided among components, such as voltage regulation or filtering.

Steady-State Behavior of Capacitors

In DC circuits, capacitors exhibit unique behaviors:

• Charging Phase: Initially, an uncharged capacitor stores charge on its plates, causing its potential difference to rise.

• Steady State: Once the capacitor’s voltage equals the supply voltage (e.g., battery voltage), no current flows through the capacitor, and it behaves as an open circuit.

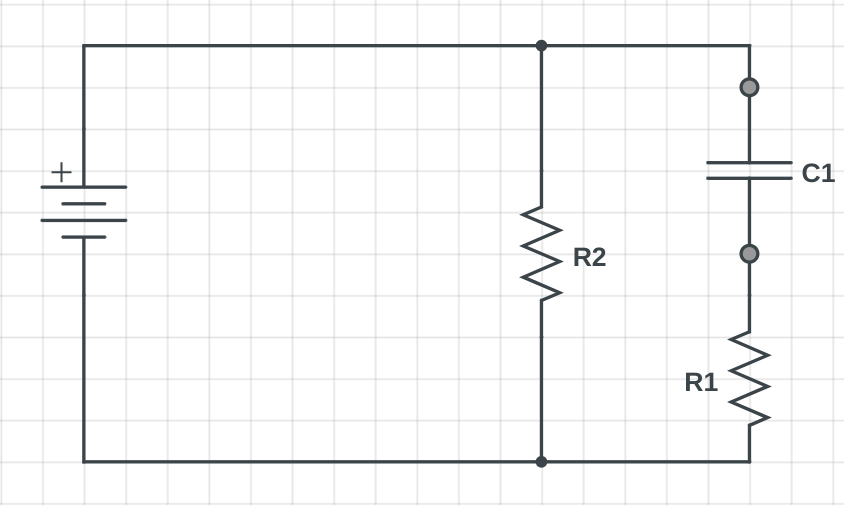
For example, in a circuit with capacitors and resistors and :

• Initially, current flows through both branches.

• As charges, less current passes through .

• At steady state, the circuit behaves as a simple series circuit with.

RC Circuits

An RC circuit is a combination of a resistor and capacitor connected in series. RC circuits are widely used in electronics for timing, filtering, and signal processing.

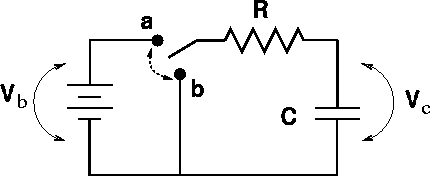
Charging the Capacitor

In an RC circuit, the capacitor charges according to the equation:

Where:

• : Charge on the capacitor at time .

• : Maximum charge the capacitor can hold.

• : Time constant, representing how quickly the capacitor charges.

The voltage across the capacitor and the current are:

Discharging the Capacitor

During discharge, the capacitor releases stored energy:

Where:

• , , and are the initial charge, voltage, and current.

The time constant determines how quickly the capacitor charges or discharges. Larger values of or slow the process, making RC circuits ideal for applications such as:

• Low-pass filters: Allowing low-frequency signals to pass while blocking high frequencies.

• High-pass filters: Allowing high-frequency signals to pass while blocking low frequencies.

Practice Problem Examples

Example 1: Voltage Distribution

Question: In a circuit with a 20V battery, a resistor , and a capacitor in parallel, determine the voltage across at and at steady state.

Solution:

• At : The capacitor has no charge, so the full voltage of the battery appears across :

• At steady state: The capacitor’s voltage equals the battery voltage. The voltage drop across is determined using Kirchhoff’s laws.

Example 2: Energy Stored in a Capacitor

Question: What is the energy stored in a 5μF capacitor with a voltage of 30V?

Solution: Energy stored in a capacitor is given by:

Substitute the values:

Key Takeaways

1. Capacitor Configurations:

   • In parallel, capacitance adds up.

   • In series, the reciprocal of capacitance adds up.

2. Steady-State Behavior:

   • Capacitors act as open circuits in DC steady-state conditions.

3. RC Circuits:

   • Charging and discharging follow exponential relationships governed by the time constant.

   • Applications include signal filtering and timing circuits.

Understanding capacitors and their applications is critical for analyzing circuits and solving problems efficiently. For more in-depth learning and practical resources, visit SlyAcademy.com, your go-to platform for physics and engineering education.