4.3 Conservation of Energy, the Work-Energy Principle, and Power

Law of Conservation of Energy

The Law of Conservation of Energy states:

The total energy in a closed system remains constant.

In a system where no external forces act, energy is conserved and can transform among:

• Potential Energy (PE)
• Kinetic Energy (KE)
• Thermal Energy (TE)

However, external forces (e.g., friction) can alter the system’s total energy.

Key Applications

• Falling objects.
• Rolling or sliding down ramps.
• Oscillating masses and springs.
• Planetary orbits.

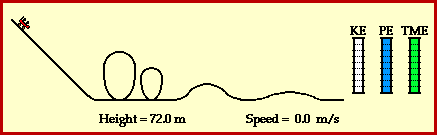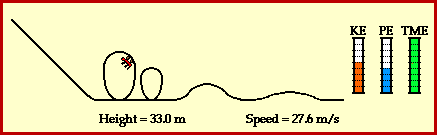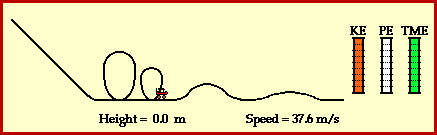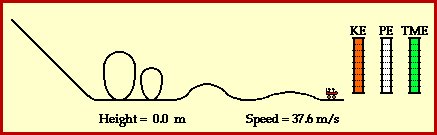
Example: Frictionless Roller Coaster

In a frictionless system, the total mechanical energy (TME)—the sum of PE and KE—is constant.

$$TME=PE+KE$$

Key Points

• Energy is conserved only when no external forces act on the system.
• Conservation applies to all forms of energy (mechanical, thermal, etc.).
• Predict and solve problems involving energy transformation and transfer using this law.

The Work-Energy Principle

The Work-Energy Principle connects work done on an object to its change in kinetic energy:

$$W=\mathrm{\Delta }KE$$

Where:

• $W$: Work done (J).
• $\Delta KE = KE_{\text{final}} – KE_{\text{initial}}$
  

Key Insights

• The principle is rooted in energy conservation.
• Applies to linear and rotational motion.
• Useful for analyzing energy changes in conservative (e.g., gravity) and non-conservative (e.g., friction) force scenarios.

Power

Power measures the rate of work or energy transfer:

$$P=\frac{W}{\mathrm{t<span\; style="font-family:\; Georgia,\; \text{'}Times\; New\; Roman\text{'},\; \text{'}Bitstream\; Charter\text{'},\; Times,\; serif;"></span>}}$$

Where:

• $P$: Power (W).
• $W$: Work done (J).
• $t$: Time (s).

Key Points About Power

• Units: Watts ($1 \, \text{W} = 1 \, \text{J/s}$).
• Scalar quantity (magnitude only).
• Indicates how quickly energy is transferred or work is done.
• Higher power means more work is done in a shorter time.

Key Takeaways

1. Energy Conservation: Total energy in a closed system remains constant; it can transform but not disappear.
2. Work-Energy Principle: Work done on an object equals its change in kinetic energy, aiding in motion analysis.
3. Power: Quantifies how quickly energy is transferred or tasks are completed.

Real-World Applications

1. Hydroelectric Dams: Convert gravitational PE to electrical energy.
2. Car Engines: Analyze work-energy transfer to optimize fuel efficiency.
3. Athletics: Measure power output in weightlifting or sprinting.