10.1 Properties of Waves

10.1 Properties of Waves

As of 2021, the College Board focuses only on Units 1-7 in the AP Physics 1 exam. However, this content on wave properties remains a valuable resource for understanding fundamental physics concepts.

Enduring Understanding 6.A 💻

A wave is a traveling disturbance that transfers energy and momentum.

Essential Knowledge 6.A.1 🏡

• Waves can propagate via different oscillation modes, such as transverse and longitudinal.

Essential Knowledge 6.A.2 🏡

• Mechanical waves require a medium for propagation.

Essential Knowledge 6.A.3 🏡

• The amplitude represents the maximum displacement of a wave from its equilibrium value.

Essential Knowledge 6.A.4 🏡

• The energy carried by a wave increases with amplitude. For example, sound waves with greater amplitudes carry more energy.

Anatomy of a Wave

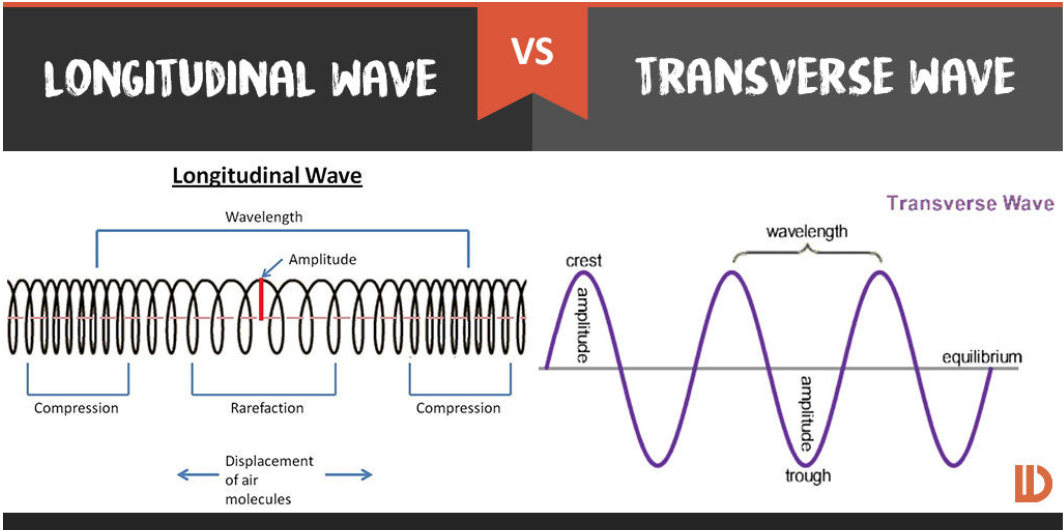
A wave is a periodic disturbance, repeating in a predictable pattern. The disturbance causes particles in the medium to oscillate either:

• Parallel to the wave’s motion (“Longitudinal waves”).

• Perpendicular to the wave’s motion (“Transverse waves”).

Key Features of Waves:

1. Amplitude:

   • The height of the wave, measured from the equilibrium position.

   • Indicates the amount of energy the wave transfers—higher amplitudes signify greater energy.

2. Wavelength:

   • The distance between repeating points of the wave.

   • For longitudinal waves: Compression to compression or rarefaction to rarefaction.

   • For transverse waves: Crest to crest or trough to trough.

3. Period:

   • The time it takes for one cycle of the wave to occur.

4. Frequency:

   • The number of wave cycles in a given time, measured in Hertz (Hz).

Velocity of a Wave

The speed of a wave depends on the medium it travels through. Mechanical waves require a medium (e.g., air, water, or a rope) and cannot propagate through a vacuum. In general:

• Waves travel faster in denser materials or materials with higher tension.

Wave Velocity Equation:

For waves:

Inverse Relationship:

If the wave’s velocity remains constant, the wavelength and frequency are inversely proportional:

• Lower frequency = Larger wavelength.

• Higher frequency = Smaller wavelength.

Practical Applications

The PhET Waves on a String simulation provides an interactive way to experiment with these properties. Adjusting the medium’s density or tension illustrates how wave velocity and energy change.

Sample Problem (AP Classroom)

Problem:

A transverse wave travels to the right along a string. Two dots, P and Q, are painted on the string. At an instant:

• Dot P has maximum displacement.

• Dot Q has zero displacement.

Tasks:

1. Indicate the instantaneous velocity and acceleration of P and Q.

2. Draw the string’s position after a time interval of , where is the period.

3. Determine the total distance traveled by dot P in one period.

Solution:

1. Velocity and Acceleration:

   • Dot P: At maximum displacement, velocity = 0; acceleration is maximum (toward equilibrium).

   • Dot Q: At equilibrium, velocity is maximum; acceleration = 0.

2. String Position at:

   • Shift each point of the wave by 1/4 of the cycle to the right.

   • Point P remains stationary since waves transfer energy, not mass.

3. Distance Traveled by Dot P:

   • In one period, dot P oscillates from maximum displacement (+A) to −A and back, covering:

Key Takeaways

• Waves transfer energy and momentum without transporting mass.

• The wave’s properties, such as amplitude, wavelength, frequency, and velocity, are interrelated.

• Understanding these properties is critical for applications in physics, engineering, and acoustics.