Energy in Modern Physics: An Overview
Introduction
Einstein’s famous equation, E=mc², revolutionized our understanding of energy and mass, illustrating their profound equivalence. In this lesson, we will explore how this principle is used to calculate the mass equivalent for a given energy transfer and vice versa. Additionally, we’ll dive into the concept of binding energy, a key metric for understanding nuclear stability, and examine two nuclear processes: fission and fusion.
Binding Energy: The Glue of Stability
Binding energy is the energy required to separate the particles in a system—such as protons and neutrons in a nucleus—from one another. It reflects the strength of the forces that hold these particles together.
Key Points about Binding Energy:
It measures the stability of a system. Systems with higher binding energy are more stable and less likely to break apart.
Binding energy is positive if energy is required to separate particles, and negative if energy is released during separation.
It is directly proportional to the mass defect, the difference between the predicted and actual mass of a nucleus.
Equation for Binding Energy:
The binding energy (BE) of a system can be calculated as:
BE = E₂ − E₁,
where:
BE = Binding energy,
E₂ = Total energy of the system,
E₁ = Energy of individual particles.
Example: Helium-4 Nucleus
Helium-4 consists of 2 protons and 2 neutrons. Its predicted mass is 4.0330 atomic mass units (u). However, the experimental mass is 4.0026 u. The difference, 0.0304 u, represents the mass defect, which can be converted into binding energy using Einstein’s equation:
E = Δm × c².
The Mass-Energy Relationship
Einstein’s special theory of relativity illustrates that mass can be converted to energy and vice versa. This relationship is quantified as:
E = mc², where:
E = Energy (Joules),
m = Mass (kg),
c = Speed of light (3.00 × 10⁸ m/s).
Energy Equivalent of 1 Atomic Mass Unit (u):
1 u = 1.6605 × 10⁻²⁷ kg, which corresponds to approximately 931.5 MeV. This conversion factor is crucial in nuclear physics for determining the energy released or required in nuclear reactions.
Binding Energy Equation: BE (MeV) = Mass Defect × 931.5 MeV/u
Average Binding Energy and Stability
The stability of a nucleus is often evaluated using its average binding energy:
Average BE = BE / A, where:
A = Mass number of the element.
Nuclei with higher average binding energy are generally more stable. For example, iron-56 has one of the highest average binding energies, making it one of the most stable elements.
Fission vs. Fusion: Harnessing Nuclear Energy
Fission: Splitting the Atom
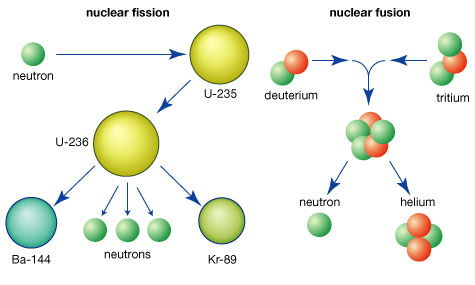
Fission is the process in which a heavy, unstable nucleus splits into two smaller nuclei upon absorbing a slow-moving neutron. This reaction releases a significant amount of energy due to the mass defect.
Key Points about Fission:
Commonly used in nuclear power plants to generate electricity.
Produces radioactive by-products that require careful handling.
Occurs naturally in some isotopes (e.g., uranium-235) and can be induced artificially.
Fusion: Powering the Stars
Fusion is the process where two light nuclei combine to form a more stable nucleus. Unlike fission, fusion does not produce long-lived radioactive by-products.
Key Points about Fusion:
Occurs naturally in stars, including the sun.
Releases more energy than fission.
Requires extremely high temperatures and pressures, making it challenging to replicate on Earth.
Comparing Fission and Fusion
| Property | Fission | Fusion |
|---|---|---|
| Definition | Splitting of a heavy nucleus | Combining of light nuclei |
| By-products | Radioactive | Non-radioactive |
| Energy Release | Moderate | High |
| Occurrence | Naturally in radioactive isotopes | Naturally in stars |
| Applications | Nuclear power plants | Experimental fusion reactors |
Practice Problems
Correct statements about the binding energy of a nucleus include which of the following?
I. It is the energy needed to separate the nucleus into its individual protons and neutrons.
II. It is the energy liberated when the nucleus is formed from the original nucleons.
III. It is the energy equivalent of the apparent loss of mass of its nucleon constituents.
Options:
A) I only
B) III only
C) I and II only
D) II and III only
E) I, II, and III
Answer: E) I, II, and III.
Which process releases more energy per reaction?
A) Fission
B) Fusion
C) Both release equal energy
D) Neither releases energy
Answer: B) Fusion.
Which element has the highest average binding energy?
A) Hydrogen
B) Uranium
C) Iron
D) Helium
Answer: C) Iron.
Applications of Binding Energy
The concept of binding energy is pivotal in various fields:
Nuclear Power: Fission reactions in nuclear reactors utilize the binding energy of uranium or plutonium isotopes to generate electricity.
Astrophysics: Fusion processes power stars, providing light and heat essential for life on Earth.
Medical Imaging and Treatments: Radioisotopes produced in nuclear reactions are used in cancer treatments and diagnostic tools.
Weapons Development: Understanding binding energy has been instrumental in the development of nuclear weapons, highlighting the need for ethical considerations in scientific advancements.







