7.6 Photoelectric Effect

Understanding the Photoelectric Effect

Introduction

The photoelectric effect is a groundbreaking phenomenon that illustrates the particle-like behavior of light. It was first explained by Albert Einstein in the early 20th century, building upon earlier work by Max Planck and others. Einstein’s explanation earned him the Nobel Prize in Physics in 1921, marking a pivotal moment in the development of quantum mechanics.

This article explores the key principles of the photoelectric effect, its underlying equations, and its applications, while also providing practice problems to reinforce your understanding.

What Is the Photoelectric Effect?

The photoelectric effect refers to the emission of electrons from a metal surface when it is exposed to light of a sufficient frequency. This phenomenon provides critical evidence for the particle nature of light, where photons transfer their energy to electrons.

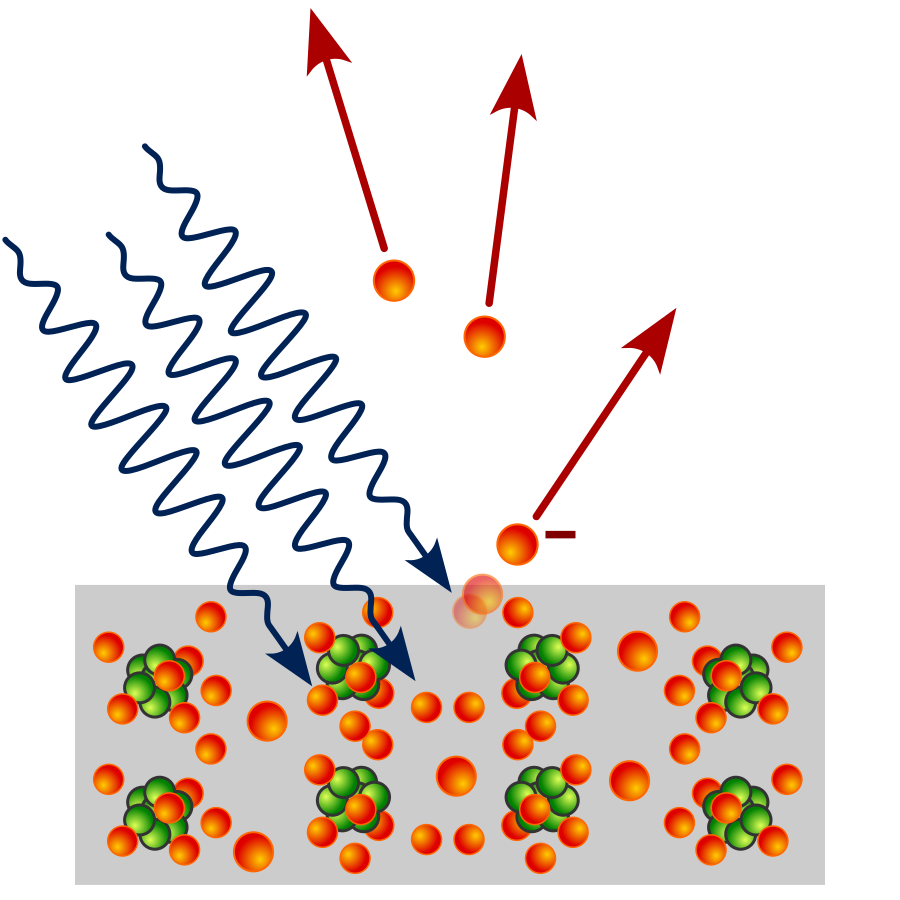
Key Principles of the Photoelectric Effect:

1. Photon Energy:

   • Light behaves as a stream of particles called photons.

   • Each photon carries energy determined by its frequency: E = hf, where:

     • E = Energy of the photon (joules),

     • h = Planck’s constant (≈6.63 × 10⁻³⁴ Js),

     • f = Frequency of the light (Hz).

2. Electron Emission:

   • Photons transfer energy to electrons in the metal surface.

   • If the photon’s energy exceeds the metal’s work function (Φ), electrons are emitted with a maximum kinetic energy: Kᵀᵀ = E – Φ, or equivalently: Kᵀᵀ = hf – Φ.

3. Threshold Frequency:

   • Electrons are only emitted if the frequency of light exceeds the threshold frequency: f_0 = Φ / h.

   • Below this frequency, no electrons are emitted, regardless of light intensity.

4. Independence from Intensity:

   • Increasing light intensity increases the number of emitted electrons but does not affect their kinetic energy. The kinetic energy depends solely on the frequency of light.

Experimental Observations

The photoelectric effect defied classical physics and validated quantum mechanics with the following observations:

1. Instantaneous Emission:

   • Electrons are emitted almost immediately after the light strikes the metal surface.

2. Intensity vs. Kinetic Energy:

   • Increasing light intensity increases the number of photoelectrons but does not affect their kinetic energy.

3. Threshold Frequency:

   • Below a certain frequency (), no electrons are emitted regardless of light intensity.

4. Work Function (Φ):

   • The work function represents the minimum energy required to free an electron from the metal surface: Φ = hf_0.

Energy Equations:

1. Photon Energy: E = hf = hc / λ, where:

   • c = Speed of light (≈3.00 × 10⁸ m/s),

   • λ = Wavelength of light (m).

2. Maximum Kinetic Energy of Electrons: Kᵀᵀ = hf – Φ.

Applications of the Photoelectric Effect

The photoelectric effect has numerous applications across various fields:

1. Solar Cells:

   • Photovoltaic cells in solar panels utilize the photoelectric effect to convert sunlight into electricity.

2. Photoelectric Detectors:

   • Used in smoke detectors, light meters, and cameras.

3. Quantum Research:

   • Provides insight into electron behavior and supports advancements in quantum mechanics.

4. Medical Imaging:

   • X-ray machines and radiation detectors exploit the principles of the photoelectric effect.

5. Lasers:

   • The phenomenon helps in understanding laser generation and operation.

Practice Problems

1. Light of a single frequency falls on a photoelectric material, but no electrons are emitted. What should be done to emit electrons?

• A) Decrease the frequency of light.

• B) Increase the frequency of light.

• C) Decrease the intensity of light.

• D) Increase the intensity of light.

Answer: B) Increase the frequency of light. To emit electrons, the light’s frequency must exceed the threshold frequency.

2. A student performs a photoelectric effect experiment and measures the maximum kinetic energy () of photoelectrons as a function of photon frequency. The data shows a straight line when plotted. What is the work function (Φ) of the material?

• A) 1.5 eV

• B) 2.0 eV

• C) 2.7 eV

• D) 4.0 eV

• E) 6.0 eV

Answer: A) 1.5 eV. From the equation , the y-intercept of the plot gives the work function.

3. Which graph best represents the relationship between maximum kinetic energy and light frequency?

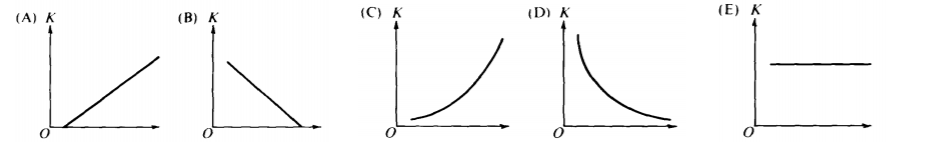
• A) A

• B) B

• C) C

• D) D

• E) E

Answer: A) Graph A. The kinetic energy increases linearly with frequency above the threshold frequency, as described by .

4. How should an experimenter increase the number of emitted electrons while reducing their kinetic energy?

• A) Increase the intensity and decrease the wavelength of light.

• B) Increase the intensity and wavelength of light.

• C) Decrease the intensity and wavelength of light.

• D) Decrease the intensity and increase the wavelength of light.

• E) None of the above.

Answer: B) Increase the intensity and wavelength of light. Increasing intensity emits more electrons, and increasing wavelength decreases their kinetic energy by reducing photon energy.