3.12 Isolines and Electric Fields

Isolines and Electric Fields

Understanding Isolines in Electric Fields

Isolines, also known as contour lines, are lines that connect points of equal value in a scalar field. In the context of electric fields, these isolines are referred to as equipotential lines.

Key Features of Equipotential Lines:

1. Definition:

   • Equipotential lines represent points of equal electric potential.

   • They are always perpendicular to electric field lines.

2. Visualization:

   • In a uniform electric field, equipotential lines appear as evenly spaced, parallel lines.

   • In a non-uniform electric field, the lines are curved.

3. Applications:

   • Equipotential lines help visualize electric potential in a region.

   • They simplify calculations involving the work done by an electric field when moving a charge between points.

4. Work and Potential Energy:

   • The work done by the electric field in moving a charged particle is equal to the change in electric potential energy.

   • Work is calculated using the difference in electric potential between two points.

Real-World Connections:

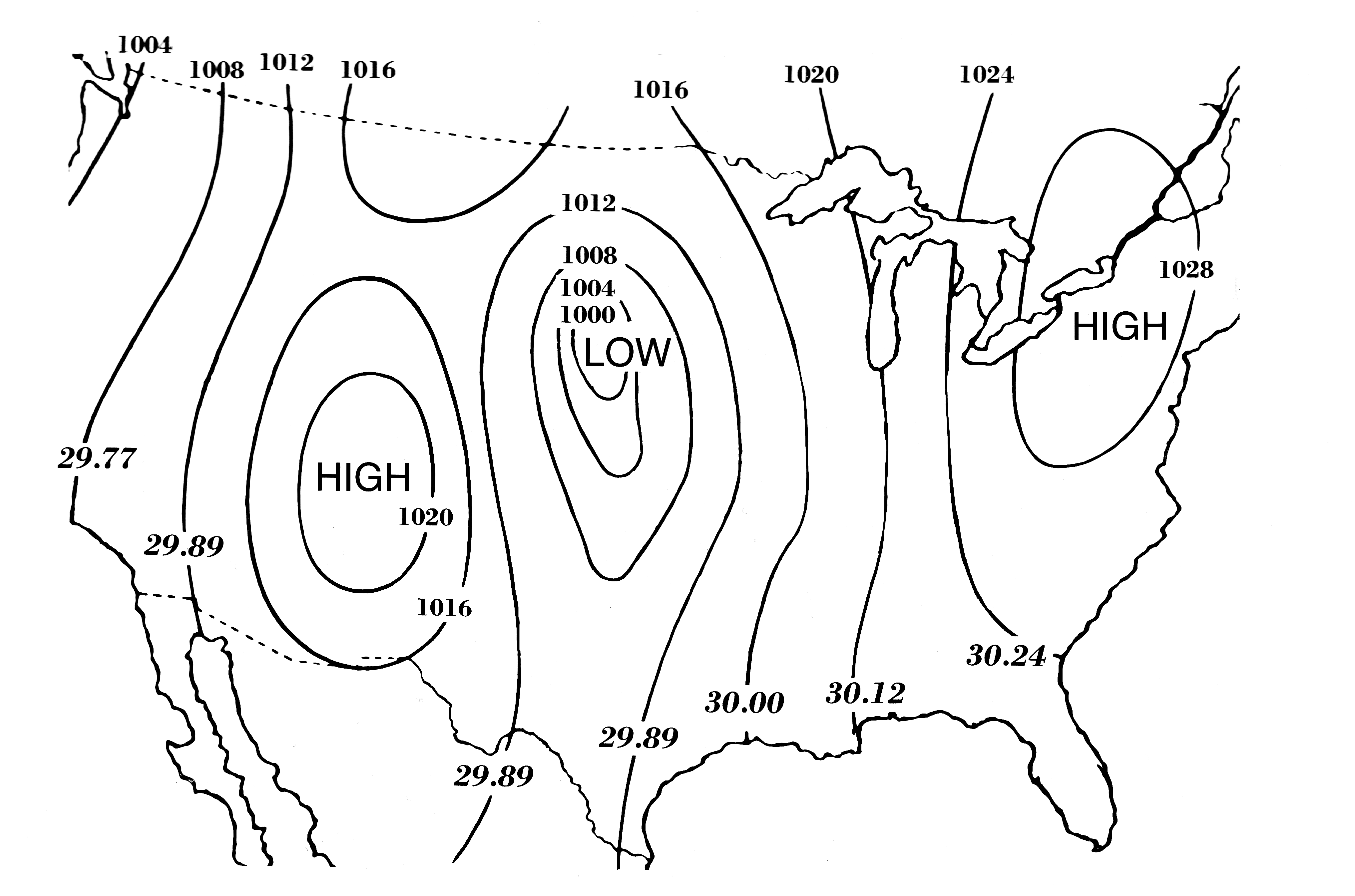
Equipotential lines are conceptually similar to contour lines on topographical maps (representing height) or barometric pressure maps (representing pressure).

Electric Potential and Voltage

Electric potential and voltage describe the potential energy of a charged particle within an electric field.

Key Points:

1. Electric Potential (“V”):

   • Represents the potential energy of a charged particle in an electric field.

   • Measured in volts (V).

   • Determined by the charge creating the field and the distance from the charge.

2. Voltage (“ΔV”):

   • Represents the difference in electric potential between two points.

   • Also measured in volts (V).

   • Example: The voltage across a battery reflects the difference in electric potential between its positive and negative terminals.

3. Key Formula: Where:

   • : Electric potential (volts).

   • : Work done (joules).

   • : Charge (coulombs).

4. Electric Potential Around a Point Charge:

   • For a point charge, , where is Coulomb’s constant, is the charge, and is the distance from the charge.

   • Equipotential lines around a point charge appear as concentric circles.

Equipotential Lines and Their Properties

Key Characteristics:

1. Perpendicular to Electric Field Lines:

   • Electric field lines intersect equipotential lines at right angles.

2. Representation of Electric Potential:

   • In uniform fields: Equipotential lines are evenly spaced and parallel.

   • In non-uniform fields: Lines are curved and vary in spacing.

3. Relationship to Work:

   • Moving a charge along an equipotential line requires no work since there is no change in potential energy.

   • Work is only done when moving a charge between lines of different potentials.

4. Direction of Electric Field:

   • Arrows representing the electric field should be perpendicular to equipotential lines and point from high potential to low potential.

Real-World Analogy:

Equipotential lines resemble contour lines on a topographic map or isobars on a weather map, with each line representing a constant value.

Example Problem

Question:

1. Direction of Electric Field at Point A:

   • The electric field points perpendicular to the equipotential line at Point A and from higher to lower potential.

2. Point of Greatest Electric Field Magnitude:

   • The electric field is strongest where equipotential lines are closest together.

3. Work Done to Move a Charge from Point C to Point E:

   • The net work is determined by the potential difference between Points C and E and the charge magnitude:

Solution:

• Calculate the potential difference .

• Multiply by the charge value to determine the work done.

Conclusion

Equipotential lines and electric fields are fundamental tools for visualizing and analyzing electric potentials and forces. By understanding their properties, we can calculate work, determine electric field directions, and better comprehend the behavior of charged particles in an electric field. These concepts are pivotal in physics and engineering applications, from circuit design to understanding natural phenomena.