3.5 Inertial vs. Gravitational Mass

Learning Targets

• Understand the difference between inertial mass and gravitational mass.
• Learn how these masses influence motion and gravitational interaction.
• Explore experiments proving the equivalence of inertial and gravitational mass.

What Is Inertial Mass?

Inertial mass measures an object’s resistance to acceleration when a force is applied. It is described by Newton’s Second Law of Motion:

$$F = ma$$

Where:

• $F$ is the force applied (N),
• $m$ is the inertial mass (kg),
• $a$ is the acceleration (m/s²).

Key Insights

• Objects with greater inertial mass require more force to accelerate.
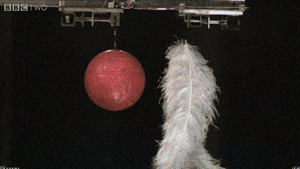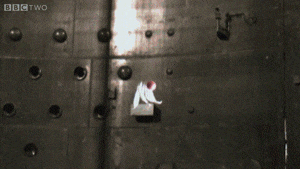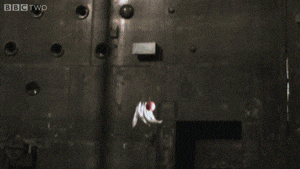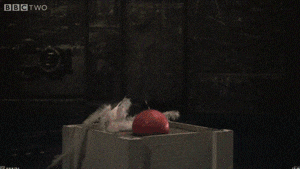
• For example, lifting a bowling ball requires more force than lifting a feather because the bowling ball has a larger inertial mass.

What Is Gravitational Mass?

Gravitational mass determines the strength of an object’s gravitational interaction with other objects. It is described by Newton’s Universal Law of Gravitation:

$$F_g = G \frac{m_1 m_2}{r^2}$$

Where:

• $F_g$ is the gravitational force (N),
• $G$ is the gravitational constant ($6.67 \times 10^{-11} \, \text{Nm}^2/\text{kg}^2$),
• $m_1$ and $m_2$ are the gravitational masses (kg),
• $r$ is the distance between the objects’ centers (m).

Key Insights

• Larger gravitational mass results in stronger gravitational attraction.
• For instance, the Earth’s larger gravitational mass creates a stronger pull on objects compared to a bowling ball.

Gravitational Mass vs. Inertial Mass

The value of inertial mass and gravitational mass is always the same for all objects. This equivalence is one of the most fundamental principles of physics and has been confirmed through various experiments.

Apollo 15 Experiment

Astronaut David Scott dropped a feather and a hammer on the Moon’s surface (where there’s no atmosphere) to demonstrate:

• Both objects hit the ground simultaneously, proving that gravitational and inertial masses are equivalent.
• In the absence of air resistance, all objects fall with the same acceleration due to gravity.

Acceleration Due to Gravity vs. Gravitational Force

• Acceleration Due to Gravity ($g$):
  Determined by the mass of the planet and the distance from its center:

  $$g = G \frac{M}{r^2}$$
  • Independent of the falling object’s mass.
  • Example: Near Earth’s surface, $g \approx 9.8 \, \text{m/s}^2$
    

• Gravitational Force ($F_g$):
  Depends on the masses of both objects and the distance between them:

  $$F_g = G \frac{m_1 m_2}{r^2}$$

Conservation of Mass

The equivalence of inertial and gravitational mass aligns with the Conservation of Mass, which states:

• The total amount of matter in a closed system remains constant, regardless of forces acting on it.

Key Takeaways

1. Inertial Mass: Measures resistance to acceleration ($F = ma$).
2. Gravitational Mass: Determines gravitational attraction strength ($F_g = G \frac{m_1 m_2}{r^2}$).
3. Equivalence Principle: Inertial and gravitational masses are equal, demonstrated by experiments like the Apollo 15 feather-drop test.
4. Acceleration Due to Gravity: Constant for all objects at a given distance from a planet, regardless of their mass.

Practice Problem

Question: A hammer and a feather are dropped in a vacuum. Which hits the ground first?

• a) Hammer
• b) Feather
• c) Both hit simultaneously
• d) Depends on their weights

Answer: c) Both hit simultaneously

Explanation: In a vacuum, gravitational acceleration is the same for all objects, regardless of mass.