5.4 Angular Momentum and Its Conservation

N
By NUM8ERS

Table of Contents

Angular Momentum and Its Conservation

Angular momentum is one of the most fundamental and fascinating concepts in physics. It governs the motion of celestial bodies, the spin of figure skaters, and even the stability of gyroscopes. Understanding angular momentum not only deepens our grasp of rotational mechanics but also unveils the underlying principles of many natural and engineered systems.

What Is Angular Momentum?

Angular momentum is the rotational equivalent of linear momentum. While linear momentum deals with objects moving in straight lines, angular momentum is concerned with objects spinning or rotating around an axis. Mathematically, angular momentum (L) is given by:

L=I⋅ωL = I \cdot \omega

Where:

  • L is the angular momentum (measured in kg⋅m2/s\text{kg} \cdot \text{m}^2/\text{s})
  • I is the moment of inertia (a measure of an object’s resistance to changes in rotation)
  • ω is the angular velocity (measured in radians per second)

Angular momentum is a vector quantity, meaning it has both magnitude and direction. The direction is determined by the right-hand rule: curl the fingers of your right hand in the direction of the object’s rotation, and your thumb will point in the direction of the angular momentum vector.

Conservation of Angular Momentum

The principle of conservation of angular momentum states:

“The total angular momentum of a system remains constant unless acted upon by an external torque.”

This fundamental law applies to all isolated systems and has profound implications in both classical and quantum physics. Here are some key takeaways about angular momentum conservation:

  1. Isolated Systems: If no external torques are present, the angular momentum before and after an event remains unchanged.
  2. Relation to Torque: Torque is the rate of change of angular momentum: τ=dLdt\tau = \frac{dL}{dt}
  3. Impact of Moment of Inertia: When an object’s moment of inertia changes, its angular velocity adjusts to maintain constant angular momentum. For instance, a figure skater pulling their arms in spins faster because their moment of inertia decreases.

Real-World Applications

1. Figure Skaters

When a figure skater pulls their arms closer to their body during a spin, they reduce their moment of inertia. To conserve angular momentum, their angular velocity increases, causing them to spin faster. Conversely, extending their arms slows them down.

2. Spinning Tops and Gyroscopes

A spinning top or gyroscope resists changes to its orientation due to the conservation of angular momentum. This principle is widely used in navigation systems, spacecraft stabilization, and robotics.

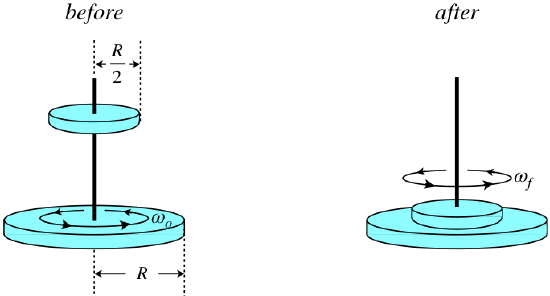
3. Colliding Disks

Consider two spinning disks on a frictionless surface. If they collide and stick together, the total angular momentum before and after the collision remains constant. This scenario highlights how angular momentum conservation governs interactions between rotating bodies.

5.4 Angular Momentum and Its Conservation

4. Planetary Orbits

Angular momentum conservation plays a crucial role in celestial mechanics. As a planet moves closer to the Sun in its elliptical orbit, its angular velocity increases to conserve angular momentum, and vice versa.

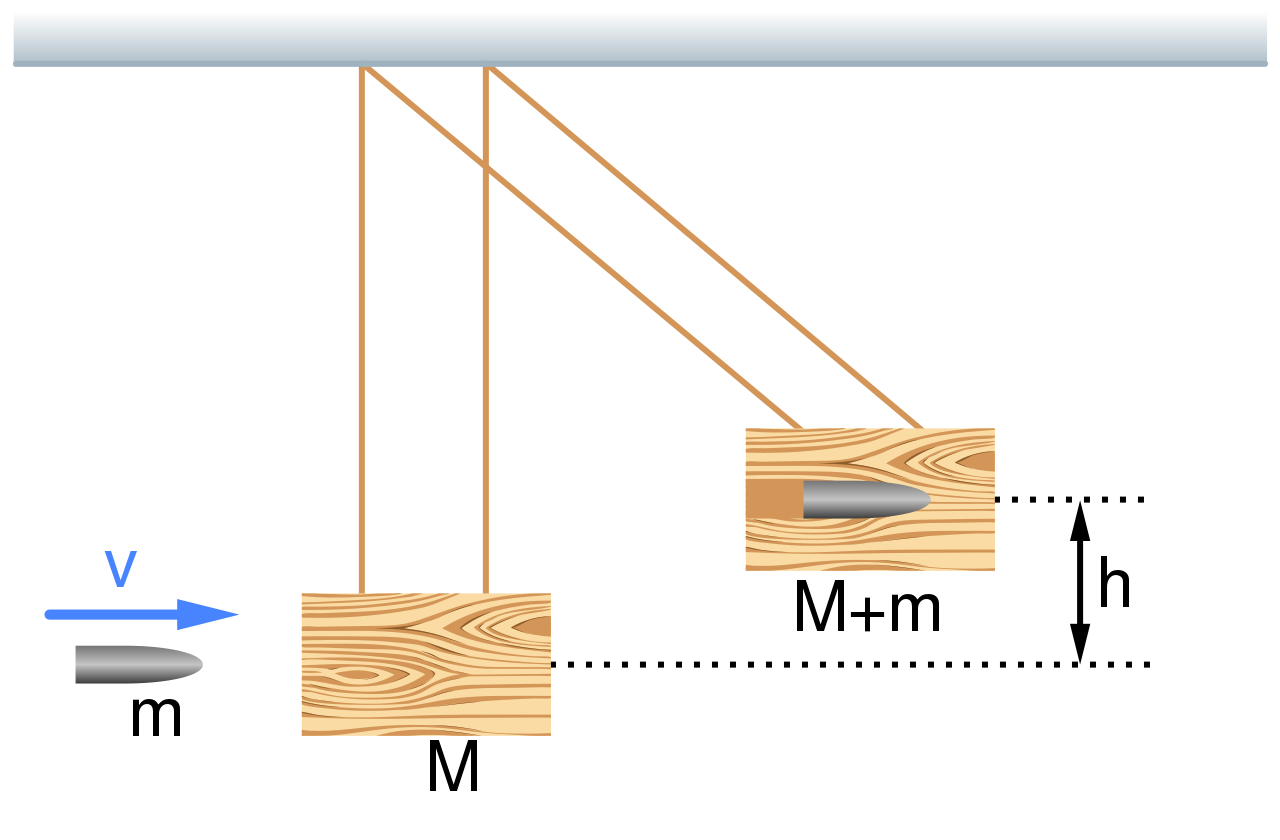
5. Ballistic Pendulum

A ballistic pendulum, used to measure projectile speeds, is a classic example of angular momentum conservation. When a bullet embeds itself into a pendulum, the combined system’s angular momentum before and after the collision remains constant. This principle helps determine the speed of the bullet based on the pendulum’s subsequent motion.

5.4 Angular Momentum and Its Conservation

Key Equations in Angular Momentum

Here are the essential equations you need to master angular momentum:

  1. Angular Momentum:

    L=I⋅ωL = I \cdot \omega

  2. Moment of Inertia for Common Shapes:

    • Solid sphere: I=25mr2I = \frac{2}{5} m r^2
    • Hollow sphere: I=23mr2I = \frac{2}{3} m r^2
    • Solid cylinder: I=12mr2I = \frac{1}{2} m r^2
    • Thin rod (about center): I=112ml2I = \frac{1}{12} m l^2

  3. Torque and Angular Momentum Relationship:

    τ=dLdt\tau = \frac{dL}{dt}

  4. Conservation of Angular Momentum:

    Linitial=LfinalL_{\text{initial}} = L_{\text{final}}

Practice Problems

1. Spinning Satellite

A satellite with a moment of inertia of 10 kg⋅m210 \, \text{kg} \cdot \text{m}^2 is spinning at 5 rad/s5 \, \text{rad/s}. If the satellite extends its solar panels, increasing its moment of inertia to 15 kg⋅m215 \, \text{kg} \cdot \text{m}^2, what is its new angular velocity?

Solution:
Using the conservation of angular momentum:

Linitial=LfinalL_{\text{initial}} = L_{\text{final}} Iinitial⋅ωinitial=Ifinal⋅ωfinalI_{\text{initial}} \cdot \omega_{\text{initial}} = I_{\text{final}} \cdot \omega_{\text{final}} 10⋅5=15⋅ωfinal10 \cdot 5 = 15 \cdot \omega_{\text{final}} ωfinal=5015=3.33 rad/s\omega_{\text{final}} = \frac{50}{15} = 3.33 \, \text{rad/s}

2. Rotating Disk Collision

Two disks collide on a frictionless surface. Disk A (I=2 kg⋅m2I = 2 \, \text{kg} \cdot \text{m}^2, ω=10 rad/s\omega = 10 \, \text{rad/s}) strikes Disk B (I=3 kg⋅m2I = 3 \, \text{kg} \cdot \text{m}^2, initially at rest). If the disks stick together, find their final angular velocity.

Solution:
Conserve angular momentum:

Ltotal=LA+LBL_{\text{total}} = L_{\text{A}} + L_{\text{B}} Lfinal=(IA+IB)⋅ωfinalL_{\text{final}} = (I_{\text{A}} + I_{\text{B}}) \cdot \omega_{\text{final}} 2⋅10+3⋅0=(2+3)⋅ωfinal2 \cdot 10 + 3 \cdot 0 = (2 + 3) \cdot \omega_{\text{final}} ωfinal=205=4 rad/s\omega_{\text{final}} = \frac{20}{5} = 4 \, \text{rad/s}

3. Ballistic Pendulum

A bullet of mass 0.01 kg0.01 \, \text{kg} traveling at 500 m/s500 \, \text{m/s} embeds itself in a pendulum of mass 2 kg2 \, \text{kg} and length 1 m1 \, \text{m}. Calculate the angular velocity of the pendulum immediately after the collision.

Solution:
Use conservation of angular momentum:

mbullet⋅vbullet⋅r=(Ipendulum+Ibullet)⋅ωm_{\text{bullet}} \cdot v_{\text{bullet}} \cdot r = (I_{\text{pendulum}} + I_{\text{bullet}}) \cdot \omega

Here, r=1 mr = 1 \, \text{m}, Ipendulum=13mpendulumr2I_{\text{pendulum}} = \frac{1}{3} m_{\text{pendulum}} r^2, and Ibullet=mbulletr2I_{\text{bullet}} = m_{\text{bullet}} r^2.

Plugging in values:

0.01⋅500⋅1=(13⋅2⋅12+0.01⋅12)⋅ω0.01 \cdot 500 \cdot 1 = \left(\frac{1}{3} \cdot 2 \cdot 1^2 + 0.01 \cdot 1^2\right) \cdot \omega
5=(23+0.01)⋅ω5 = \left(\frac{2}{3} + 0.01\right) \cdot \omega
ω=50.6767=7.39 rad/s\omega = \frac{5}{0.6767} = 7.39 \, \text{rad/s}

Conclusion

Angular momentum and its conservation offer powerful tools for understanding rotational systems, from everyday objects to celestial mechanics. By mastering these principles, you gain insights into a world governed by symmetry, conservation, and the elegance of rotational motion. Practice applying these concepts to enhance your physics prowess and unravel the mysteries of spinning systems!

5.4 Angular Momentum and Its Conservation

Angular Momentum and Its Conservation FAQs

1. What is angular momentum?

Angular momentum is a vector quantity that represents the rotational equivalent of linear momentum. It is given by: where:

  • : Angular momentum,

  • : Moment of inertia,

  • : Angular velocity.

2. How is angular momentum calculated for a particle?

For a particle, angular momentum is calculated as: where:

  • : Position vector from the axis of rotation,

  • : Linear momentum of the particle,

  • : Cross product.

3. What are the units of angular momentum?

The SI unit of angular momentum is .

4. What is the moment of inertia?

The moment of inertia () quantifies an object’s resistance to rotational acceleration. It depends on the mass distribution and axis of rotation: where is the distance of mass from the axis.

5. What is the principle of conservation of angular momentum?

The total angular momentum of a closed system remains constant if no external torque acts on it:

6. How does angular momentum differ from linear momentum?

  • Angular momentum: Describes rotational motion.

  • Linear momentum: Describes straight-line motion. Both are conserved in isolated systems.

7. How does torque affect angular momentum?

Torque is the rate of change of angular momentum:

8. What is the relationship between torque and angular momentum?

If net external torque is zero, angular momentum remains constant. Otherwise, torque changes angular momentum over time.

9. How is angular momentum conserved in rotational motion?

In the absence of external torque, an object’s angular momentum remains unchanged, even if its moment of inertia changes.

10. What is an example of conservation of angular momentum?

Figure skaters pulling their arms in to spin faster is a classic example. Reducing their moment of inertia increases their angular velocity while conserving angular momentum.

11. How is angular momentum conserved in planetary motion?

Planets conserve angular momentum as they orbit the sun. When closer to the sun, their speed increases to maintain constant angular momentum.

12. What are the components of angular momentum?

Angular momentum has two components:

  1. Orbital angular momentum: Due to motion around a central axis.

  2. Spin angular momentum: Due to an object’s rotation about its own axis.

13. What is the significance of angular momentum in astronomy?

Angular momentum explains the rotation of celestial bodies, formation of galaxies, and orbital dynamics of planets and moons.

14. How is angular momentum related to rotational kinetic energy?

Rotational kinetic energy is expressed as: where is angular momentum and is moment of inertia.

15. How does the axis of rotation affect angular momentum?

The axis of rotation determines the direction of angular momentum, as it depends on the cross product of position and linear momentum vectors.

16. How is angular momentum conserved in collisions?

In collisions involving rotational motion, if no external torque acts, the total angular momentum before and after the collision remains constant.

17. What is the angular momentum of a rigid body?

For a rigid body rotating about a fixed axis: where is the moment of inertia and is angular velocity.

18. How does angular momentum apply to gyroscopes?

Gyroscopes maintain their orientation due to angular momentum conservation, resisting changes to their rotational axis.

19. How does angular momentum affect precession?

Precession is the slow conical motion of a rotating object’s axis, caused by external torques acting on its angular momentum.

20. What is the angular momentum of a point particle?

For a point particle with linear momentum at a distance from the axis: where is the angle between and .

21. How is angular momentum used in quantum mechanics?

In quantum mechanics, angular momentum is quantized and described by operators, determining the properties of particles and atoms.

22. How does angular momentum relate to torque in rotational equilibrium?

In rotational equilibrium, the net torque is zero, ensuring angular momentum remains constant.

23. What is the angular momentum of a rotating disk?

For a uniform disk rotating about its center: where is mass, is radius, and is angular velocity.

24. How is angular momentum conserved in the formation of galaxies?

During galaxy formation, collapsing gas clouds conserve angular momentum, leading to faster rotation and flattened disk shapes.

25. What is the relationship between angular velocity and angular momentum?

Angular momentum is proportional to angular velocity for a given moment of inertia:

26. How is angular momentum conserved in sports?

In diving or gymnastics, athletes adjust their body positions to change their moment of inertia, conserving angular momentum while altering spin rates.

27. How does angular momentum apply to spinning tops?

Spinning tops resist changes to their orientation due to angular momentum, stabilizing their motion despite gravitational torque.

28. How does angular momentum affect satellite orbits?

Satellites conserve angular momentum, maintaining stable orbits and orientation unless external torques act on them.

29. What is the angular momentum of a rotating sphere?

For a solid sphere rotating about its diameter:

30. How is angular momentum conserved in explosions?

In explosions, the total angular momentum of the fragments equals the angular momentum of the system before the explosion, provided no external torque acts.

31. How does angular momentum apply to rotating black holes?

Rotating black holes, described by the Kerr metric, conserve angular momentum as they form and interact with surrounding matter.

32. How does friction affect angular momentum?

Friction exerts torque, leading to a change in angular momentum and eventually slowing down rotation.

33. How is angular momentum calculated in circular motion?

For a particle in uniform circular motion: where is tangential velocity and is the radius.

34. How is angular momentum conserved in rocket propulsion?

In rocket propulsion, the angular momentum of expelled gases is equal and opposite to the angular momentum of the rocket, conserving total angular momentum.

35. What is the angular momentum of Earth?

Earth’s angular momentum comes from its rotation about its axis and its orbital motion around the sun, both of which are conserved.

36. How does angular momentum apply to star formation?

As a star forms, conservation of angular momentum causes it to spin faster as its size decreases.

37. What is spin angular momentum?

Spin angular momentum is the intrinsic angular momentum of particles, unrelated to motion in space, significant in quantum mechanics.

38. How does angular momentum affect stability in vehicles?

Angular momentum of rotating wheels (gyroscopic effect) enhances vehicle stability, particularly in bicycles and motorcycles.

39. What is the role of angular momentum in tidal locking?

Angular momentum transfer between a planet and its moon leads to tidal locking, where the moon always shows the same face to the planet.

40. How is angular momentum used in wind turbines?

Wind turbines conserve angular momentum as their blades rotate, converting wind energy into mechanical energy.

41. What is the effect of changing the axis of rotation on angular momentum?

Changing the axis of rotation alters the direction of angular momentum but conserves its magnitude if no external torque acts.

42. How does angular momentum relate to energy conservation?

Angular momentum conservation complements energy conservation, ensuring consistent rotational motion in isolated systems.

43. How does angular momentum explain figure skaters’ spins?

Skaters pull their arms inward to decrease moment of inertia, increasing angular velocity while conserving angular momentum.

44. What is the relationship between angular momentum and precession?

Precession occurs when torque acts on angular momentum, causing the axis of rotation to trace a cone-shaped path.

45. How does angular momentum affect disk drives?

Disk drives rely on conservation of angular momentum for stable and consistent rotation, critical for data storage.

46. How is angular momentum conserved in particle physics?

In particle interactions, angular momentum conservation helps predict outcomes, such as spin states and scattering angles.

47. How does angular momentum apply to robotics?

Robots use angular momentum control for stability and precision in movements, especially for humanoid and balancing robots.

48. How is angular momentum conserved in rotating galaxies?

Galaxies conserve angular momentum, maintaining their rotational dynamics and structure over time.

49. What is the significance of angular momentum in engineering?

Angular momentum principles guide the design of rotating machinery, gyroscopes, and stabilization systems in engineering.

50. Why is understanding angular momentum and its conservation important?

Understanding angular momentum and its conservation is crucial for solving problems in mechanics, astronomy, quantum physics, and engineering, ensuring accurate predictions and efficient designs.

SlyAcademy.com

AP Physics C: Mechanics

Angular Momentum and Its Conservation Wikipedia

N
Leave a comment
Your email address will not be published. Required fields are marked *

Choose Topic

Recent Comments

No comments to show.
Related
N
NUM8ERS
0
0
5.4 Angular Momentum and Its Conservation
December 13, 2024
Save
5.4 Angular Momentum and Its Conservation
N
NUM8ERS
0
0
5.3 Rotational Dynamics and Energy
December 13, 2024
Save
5.3 Rotational Dynamics and Energy
N
NUM8ERS
0
0
5.2 Rotational Kinematics
December 13, 2024
Save
5.2 Rotational Kinematics
N
NUM8ERS
0
0
5.1 Torque and Rotational Statics
December 13, 2024
Save
5.1 Torque and Rotational Statics
More By Author
N
NUM8ERS
0
0
Unit 5 Overview: Writing Classes
December 31, 2024
Save
Unit 5 Overview: Writing Classes
N
NUM8ERS
0
0
4.5 Informal Code Analysis
December 31, 2024
Save
4.5 Informal Code Analysis
N
NUM8ERS
0
0
4.4 Nested Iteration
December 31, 2024
Save
4.4 Nested Iteration
N
NUM8ERS
0
0
4.3 Developing Algorithms Using Strings
December 31, 2024
Save
4.3 Developing Algorithms Using Strings