5.2 Rotational Kinematics
Introduction
Rotational kinematics is a fascinating branch of mechanics that deals with the motion of objects rotating around an axis. Just as translational kinematics describes objects moving linearly, rotational kinematics focuses on angular displacement, angular velocity, and angular acceleration. These concepts help us understand how wheels spin, how the Earth rotates, and even how galaxies move.
In this unit, we will explore the principles of rotational kinematics and how they compare to their translational counterparts. We’ll dive into the equations, learn about the relationships between angular and linear variables, and solve practical problems to solidify our understanding. This comprehensive guide will ensure that students have a deep and intuitive grasp of rotational kinematics.
Translational vs. Rotational Kinematics
Objects in the physical world often exhibit both translational and rotational motion. To understand rotational kinematics, it is essential to first compare it to translational kinematics. Here are the primary variables used in both:
| Translational Motion | Rotational Motion |
|---|---|
| Displacement (Δx) | Angular Displacement (Δθ) |
| Velocity (v) | Angular Velocity (ω) |
| Acceleration (a) | Angular Acceleration (α) |
Key Equations
| Translational Kinematics | Rotational Kinematics |
| v = v0 + at | ω = ω0 + αt |
| Δx = v0t + ½at2 | Δθ = ω0t + ½αt2 |
| v2 = v02 + 2aΔx | ω2 = ω02 + 2αΔθ |
Analogous Concepts
Displacement (Δx) vs. Angular Displacement (Δθ): Angular displacement measures how much an object rotates, typically expressed in radians.
Velocity (v) vs. Angular Velocity (ω): Angular velocity represents the rate of change of angular displacement.
Acceleration (a) vs. Angular Acceleration (α): Angular acceleration measures how quickly angular velocity changes.
Both systems share time as a common variable, allowing us to link linear and rotational motion when necessary.
Connecting Rotational and Linear Motion
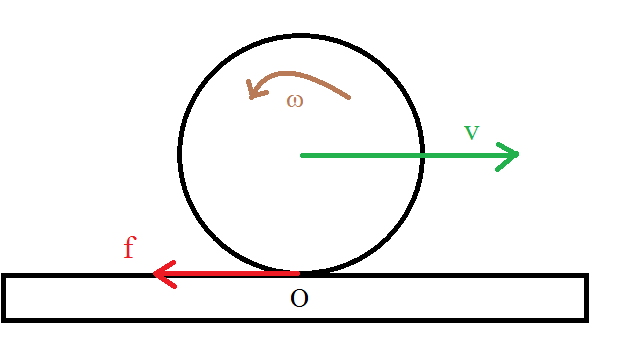
When objects exhibit rolling motion, their translational and rotational motions are intertwined. This occurs without slippage, where the point of contact is momentarily stationary relative to the surface. Here are the key relationships:
Linear and Angular Displacement:
Linear and Angular Velocity:
Linear and Angular Acceleration:
These equations provide a powerful way to transition between linear and rotational variables, making it easier to analyze systems where both motions occur simultaneously.
Real-World Applications of Rotational Kinematics
1. Rotating Wheels
Consider a bicycle wheel spinning around its hub. The wheel’s rotational motion translates to linear motion that propels the bicycle forward. Using the above equations, we can calculate the speed of the bicycle based on the angular velocity of its wheels.
2. Satellite Orbits
Satellites often rotate while orbiting planets. Understanding their angular velocity and acceleration is critical for maintaining stable orbits and ensuring proper alignment for communication.
3. Sports
From the spinning motion of a basketball to the angular acceleration of a gymnast, rotational kinematics is fundamental in analyzing and optimizing athletic performance.
Solving Rotational Kinematics Problems
Let’s apply our understanding of rotational kinematics through practical examples.
Example 1: Freight Train Acceleration
Problem: A freight train’s 0.350-m-radius wheels have an angular acceleration of 0.250 rad/s². After 200 revolutions (assuming no slippage), determine:
(a) How far the train has moved down the track.
(b) The final angular velocity of the wheels and the linear velocity of the train.
Solution:
Angular Displacement (Δθ):
Linear Displacement (Δx):
Final Angular Velocity (ω): Using ω2 = ω02 + 2αΔθ:
Linear Velocity (v):
Example 2: Yo-Yo Angular Acceleration
Problem: A yo-yo has a center shaft radius of 0.250 cm. If its string is stationary and the yo-yo accelerates away at 1.50 m/s², determine:
(a) The angular acceleration of the yo-yo.
(b) Its angular velocity after 0.750 s if it starts from rest.
Solution:
Angular Acceleration (α):
Angular Velocity (ω): Using ω = ω0 + αt:
Practice Questions
A disc with a radius of 0.5 m accelerates from rest with an angular acceleration of 2 rad/s². Calculate:
The angular displacement after 4 seconds.
The linear displacement of a point on the edge of the disc.
A spinning top has an angular velocity of 30 rad/s. If it decelerates at 5 rad/s², how long will it take to come to a stop?
A tire rotates at a constant angular velocity of 10 rad/s. If its radius is 0.3 m, calculate the linear speed of a point on its edge.
Conclusion
Rotational kinematics provides a robust framework for understanding the motion of rotating objects. By drawing parallels with translational kinematics, we can approach problems with confidence and clarity. Whether analyzing a spinning wheel, a rolling yo-yo, or a celestial body, the principles of rotational kinematics offer invaluable insights into the mechanics of the physical world.
With practice and application, students can master these concepts and confidently tackle real-world challenges involving rotational motion.
Rotational Kinematics FAQs
1. What is rotational kinematics?
Rotational kinematics describes the motion of objects that rotate around an axis, using angular displacement, angular velocity, and angular acceleration.
2. What is angular displacement?
Angular displacement is the angle through which an object rotates about a fixed axis. It is measured in radians (rad), degrees (°), or revolutions.
3. What is the formula for angular displacement?
Angular displacement () is given by: where:
: Final angle,
: Initial angle.
4. What is angular velocity?
Angular velocity () is the rate of change of angular displacement over time:
5. What are the units of angular velocity?
The SI unit of angular velocity is radians per second (rad/s).
6. What is angular acceleration?
Angular acceleration () is the rate of change of angular velocity over time:
7. What are the units of angular acceleration?
The SI unit of angular acceleration is radians per second squared (rad/s).
8. How are angular velocity and angular acceleration related?
Angular acceleration is the derivative of angular velocity with respect to time:
9. What is the difference between linear and angular kinematics?
Linear kinematics: Describes motion along a straight line.
Angular kinematics: Describes motion about a rotational axis.
10. How is angular velocity related to linear velocity?
Linear velocity () is related to angular velocity by: where is the radius of the circular path.
11. What is the equation for angular displacement in terms of angular velocity and time?
For constant angular velocity:
12. How is rotational motion represented graphically?
Rotational motion is often represented with graphs of angular displacement, angular velocity, and angular acceleration versus time.
13. What are the kinematic equations for rotational motion?
The kinematic equations for constant angular acceleration are:
14. What is the angular equivalent of Newton’s first law?
An object remains in rotational equilibrium (no angular acceleration) unless acted upon by an external torque.
15. How is angular acceleration related to torque?
Torque () and angular acceleration are related by: where is the moment of inertia.
16. What is the relationship between angular displacement and arc length?
Arc length () is related to angular displacement by: where is the radius of the circle.
17. What is the role of radians in rotational kinematics?
Radians are the standard unit for measuring angular displacement because they directly relate the arc length to the radius:
18. How is angular velocity converted to revolutions per minute (RPM)?
To convert angular velocity from rad/s to RPM:
19. What is the relationship between angular and tangential acceleration?
Tangential acceleration () is related to angular acceleration by:
20. How is centripetal acceleration related to angular velocity?
Centripetal acceleration () is given by:
21. What is the moment of inertia in rotational motion?
Moment of inertia () quantifies an object’s resistance to changes in rotational motion. It depends on mass distribution and the axis of rotation.
22. How does radius affect angular velocity?
Angular velocity is independent of radius, but linear velocity increases with radius for the same angular velocity.
23. What are some examples of rotational motion in daily life?
Spinning wheels.
Rotating fans.
Earth’s rotation.
Wind turbines.
24. How does angular velocity vary in uniform circular motion?
In uniform circular motion, angular velocity remains constant because the object rotates at a steady rate.
25. How is rotational energy calculated?
Rotational kinetic energy is:
26. How do torque and angular acceleration relate in kinematics?
Torque causes angular acceleration according to the equation:
27. What is the significance of angular kinematics in engineering?
Angular kinematics helps design rotating machinery, analyze vehicle dynamics, and optimize motion in mechanical systems.
28. How does angular velocity affect satellites?
Satellites maintain stable orbits and orientations by controlling angular velocity and using gyroscopic principles.
29. How does angular displacement relate to oscillatory motion?
In oscillatory motion, angular displacement describes the periodic rotation about an equilibrium position, such as in pendulums.
30. What is the relationship between angular and linear acceleration?
Linear acceleration is the tangential counterpart of angular acceleration:
31. How do angular quantities change with time in rotational kinematics?
Angular quantities like displacement, velocity, and acceleration follow specific kinematic equations for uniform or variable angular motion.
32. What is angular frequency?
Angular frequency () is the rate of rotation in radians per second. It is related to frequency () by:
33. How is angular velocity measured?
Angular velocity is measured using tools like tachometers or by calculating the change in angular displacement over time.
34. What is angular momentum?
Angular momentum () is the rotational analog of linear momentum:
35. What is rotational inertia?
Rotational inertia is another term for moment of inertia, indicating an object’s resistance to angular acceleration.
36. How do rotational kinematics apply to gyroscopes?
Gyroscopes use principles of angular momentum and rotational kinematics to maintain orientation and stability.
37. What is the angular equivalent of distance, velocity, and acceleration?
Distance: Angular displacement ().
Velocity: Angular velocity ().
Acceleration: Angular acceleration ().
38. How does angular velocity affect rotational energy?
Rotational energy increases with the square of angular velocity:
39. How is rotational kinematics used in robotics?
Rotational kinematics helps control joint motions, optimize robotic arm trajectories, and analyze rotational dynamics in robots.
40. What is the role of angular displacement in gear systems?
Angular displacement determines the relative rotation between interconnected gears, affecting speed and torque transmission.
41. How does angular velocity vary in non-uniform circular motion?
In non-uniform circular motion, angular velocity changes due to angular acceleration, leading to varying rotational speeds.
42. What is the angular analog of work and power?
Work: .
Power: .
43. How does rotational motion affect stability?
Stable rotational motion depends on maintaining low angular acceleration and balancing torques around the axis of rotation.
44. How is rotational kinematics applied in vehicles?
Rotational kinematics analyzes wheel rotation, drivetrain dynamics, and engine performance to improve efficiency and safety.
45. How does angular velocity affect aerodynamics?
Angular velocity influences the stability and control of rotating systems like propellers, turbines, and airplane wings.
46. How do rotational kinematics relate to pendulums?
In pendulums, angular displacement, velocity, and acceleration describe the motion as it swings around a pivot point.
47. How is rotational motion analyzed in celestial bodies?
Rotational kinematics explains phenomena like planetary rotation, tidal locking, and the angular motion of stars and galaxies.
48. How do frictional forces affect rotational kinematics?
Frictional forces create torque, which can slow down or stop rotational motion, influencing angular acceleration.
49. What is the role of angular acceleration in spinning tops?
Angular acceleration affects the stability and precession of spinning tops, maintaining their upright motion.
50. Why is understanding rotational kinematics important?
Understanding rotational kinematics is crucial for analyzing mechanical systems, improving engineering designs, and solving real-world problems involving rotation.