1.3 Point Charges Fields & Potentials

Point Charges Fields & Potentials

Point Charges: Electric Fields & Potentials

Electric Fields & Work

Bringing two like charges close together or separating two opposite charges requires work. This principle connects to concepts from mechanics, where work is defined as:

or, using calculus:

Here, is the work done, is the force applied, and is the displacement over which the force is applied. Applying this to Coulomb’s Law gives:

Where:

• : Change in electric potential energy.

• : Coulomb’s constant.

• : Charges involved.

• : Distance between charges.

Electric potential energy is a scalar quantity, meaning it has magnitude but no direction. For a system of multiple charges, the total is the sum of the individual potential energies, considering both positive and negative contributions.

Expressing in Terms of Electric Field Strength

The electric potential energy can also be expressed using electric field strength:

Here, is equivalent to the change in electric potential. Thus, the work can be calculated as:

Review Note: The principle of conservation of energy applies here—the total energy remains constant.

Potential Difference

The potential difference quantifies the work done per unit charge to move a charge in an electric field:

Voltage is a scalar quantity and is expressed as Joules per Coulomb (J/C). For point charges, voltage is determined by:

Where is the source charge and is the distance from the charge. As approaches infinity, approaches zero, indicating no electric potential influence from the charge.

For systems with multiple point charges, the total potential is the sum of the potentials from each charge:

Conservative Nature of Electric Fields

Electric fields are conservative fields, meaning the work done to move a charge depends only on the initial and final positions, not the path taken. The work done in moving a charge corresponds to changes in electric potential energy.

Caution:

• (electric potential energy) is measured in Joules.

• (potential difference) is measured in Joules per Coulomb (J/C).

Electric Potential of a Point Charge

For a single positive charge, the electric potential decreases as the distance from the charge increases. Equipotential lines around the charge form concentric circles, and electric field lines intersect these equipotential lines at right angles.

Equipotential Lines

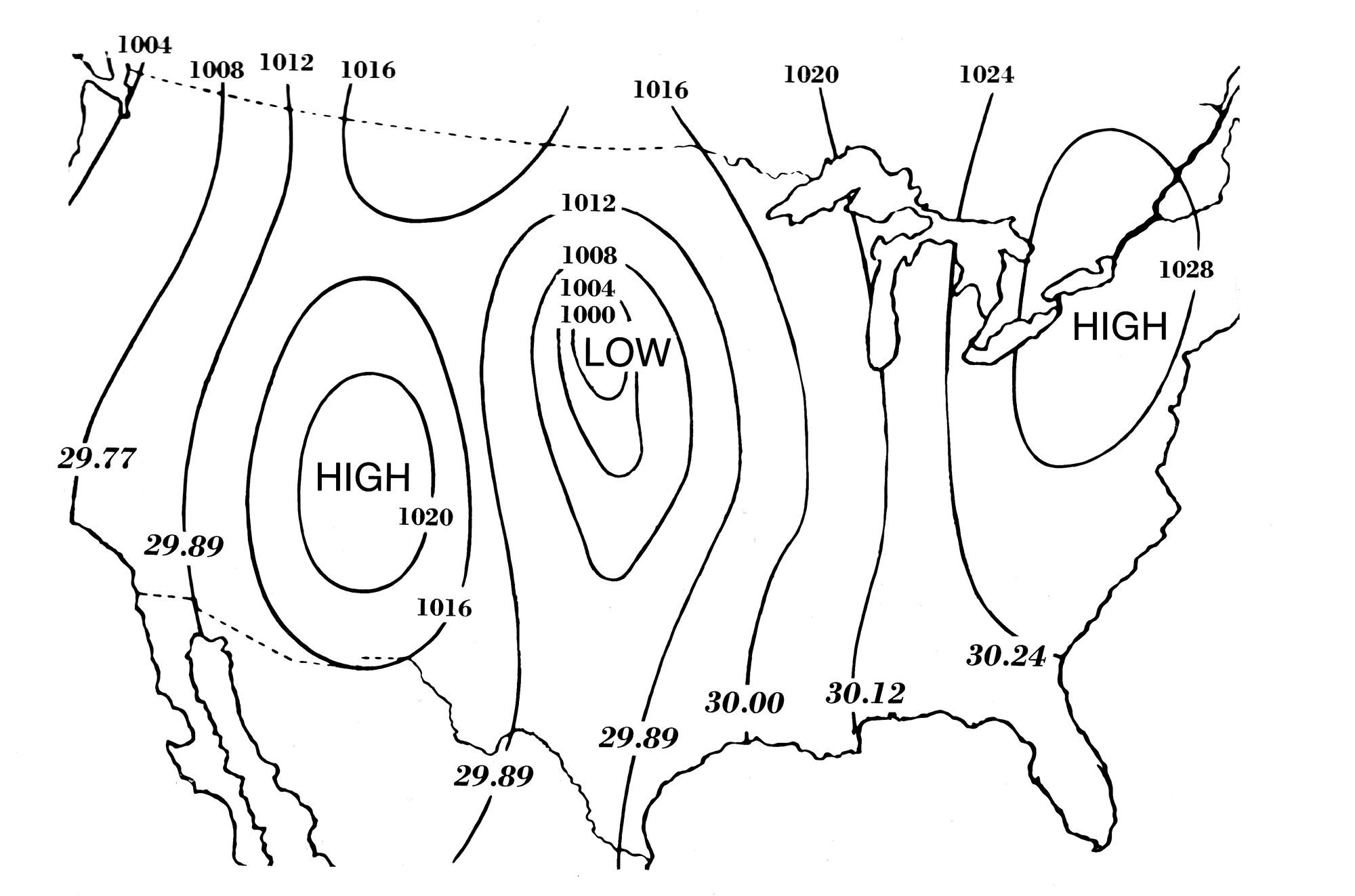
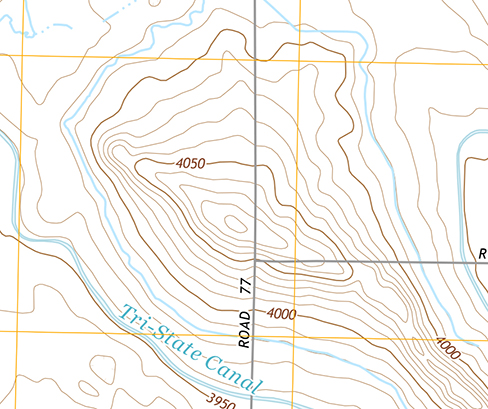
Equipotential lines represent areas with the same potential, akin to contour lines on a topographical map or isobars on a weather map. These lines simplify calculations by allowing us to focus on potential differences without considering the details of field strength.

Calculating Potential Difference

To find the potential difference between two points and :

This integral calculates the work required to move a charge between two positions within an electric field.

Applications of Equipotential Lines

1. Mapping Electric Fields:

   • Equipotential lines provide a visual representation of voltage around a charge.

   • Field lines (electric field vectors) always intersect equipotential lines perpendicularly.

2. Topographical Analogy:

   • Equipotential lines in electric fields are analogous to contour lines on a map, representing equal elevation.

3. Simplifying Calculations:

   • Movement along an equipotential line requires no work, as the potential difference is zero.

Practice Questions

1. Direction of Electric Field Lines

Question: Describe the direction of the electric field at a point relative to equipotential lines.

Answer: Electric field lines point from high potential to low potential and are perpendicular to equipotential lines. For example, at a given point , the electric field may point up and to the right, aligning with the potential gradient.

2. Field Strength and Potential

Question: At which point is the electric field strongest?

Answer: The electric field strength is greatest where equipotential lines are closest together, indicating the largest potential gradient.

3. Work and Potential Energy

Question: How much net work must be done to move a charge from point to point along equipotential lines?

Answer: Since work is related to potential difference: If the potential difference is known, multiply by the charge to find the work done.

4. Graphical Representation of Field Strength

Question: Analyze the graph showing field strength variation.

Answer: At points where the potential lines converge or diverge rapidly, the field strength changes significantly. Identify these points to determine where the field is strongest or weakest.