1.2 Electric Fields & Electric Potential

Electric Fields & Electric Potential: A Comprehensive Guide

Understanding Electric Fields

Every charged object creates an electric field around it, much like how objects with mass create gravitational fields. The difference, however, lies in the behavior: while gravitational fields are always attractive, electric fields can be either attractive or repulsive. To visualize these fields, we use field lines to represent the direction a positive test charge would move in the field.

Rules for Drawing Electric Fields:

1. Field lines are vectors and must include arrows indicating direction.

2. Lines radiate outward from positive charges and inward toward negative charges.

3. The strength of the field is depicted by the density of the field lines—closer lines represent stronger fields.

4. Field lines must never touch or cross; overlapping lines would signify an infinitely strong field, which is physically impossible.

Types of Electric Fields

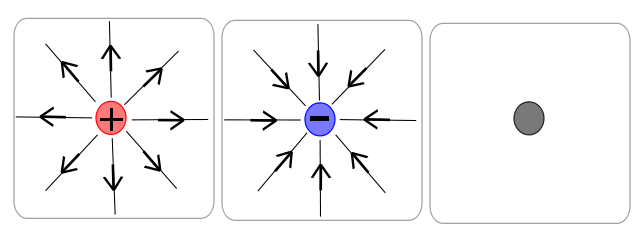
1. Point Charges

Electric fields around point charges exhibit radial symmetry, with lines radiating outward for positive charges and inward for negative charges.

2. Two Point Charges

When two charges are in proximity, the field lines illustrate the interaction between them. For like charges, lines repel each other; for opposite charges, lines converge.

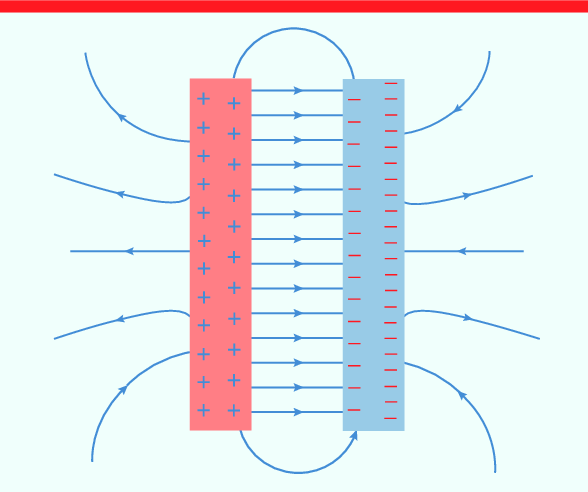
3. Parallel Plate Fields

Between two parallel plates with opposite charges, the field is uniform, meaning the field strength is constant throughout the region.

Electric Field Strength

The strength of an electric field, denoted by , can be calculated using:

Where:

• : Electric field strength (N/C).

• : Electrostatic force experienced by a test charge.

• : Magnitude of the test charge.

Alternatively, for point charges:

Where:

• Nm²/C² (Coulomb’s constant).

• : Charge creating the field.

• : Distance from the charge.

Superposition of Electric Fields

To find the net electric field at a point, combine individual field vectors using methods such as:

1. Head-to-Tail Method: Place the tail of one vector at the head of another and draw a resultant vector.

2. Parallelogram Method: Place vectors with the same origin and draw the diagonal of the parallelogram formed.

Electric Fields in Conductors and Insulators

Conductors:

In conductors, free electrons move to counteract any internal electric field, leaving the field strength inside a conductor at zero. Charges reside only on the surface.

A classic example of this phenomenon is the Faraday Cage, where an external electric field induces charges on the conductor’s surface, canceling the field inside.

Insulators:

In insulators, charges do not move freely. However, the presence of an electric field can cause polarization, where positive and negative charges within the material separate slightly, creating internal dipoles.

Electric Potential

Electric potential represents the work required to move a unit positive charge from infinity to a point in the field. It is related to electric field strength by:

Where:

• : Electric potential (volts).

• : Source charge.

• : Distance from the charge.

Applications of Electric Fields

1. Charged Rod and Water Stream

A charged rod brought near a stream of water bends the stream, as the field interacts with the polar water molecules.

2. Static Balloons

A charged balloon attracts neutral objects through polarization, demonstrating the principles of electric fields.

3. Faraday Cages

Used in shielding sensitive electronics, Faraday cages block external electric fields by redistributing charges on their surface.

Practice Problems

1. Ranking Electric Forces

Question: In a uniform electric field, rank the forces experienced by identical charges placed at different locations.

Answer: The force is the same at all locations, as the field strength is uniform, and force depends linearly on charge.

2. Zero Electric Field Strength

Question: Where is the electric field strength zero between a positive charge and a negative charge?

Answer: The field strength is zero at a point closer to the smaller charge because the larger charge exerts a stronger field. Specifically, the point lies twice as far from as from.

3. Electric Field Graphs

Question: Which graph correctly represents electric field strength between two charges?

Answer: Graph A is correct. Field strength trends toward infinity near the charges and is zero where forces from the charges cancel.