Cross Sections Perpendicular to the y-axis (SQUARE) Free
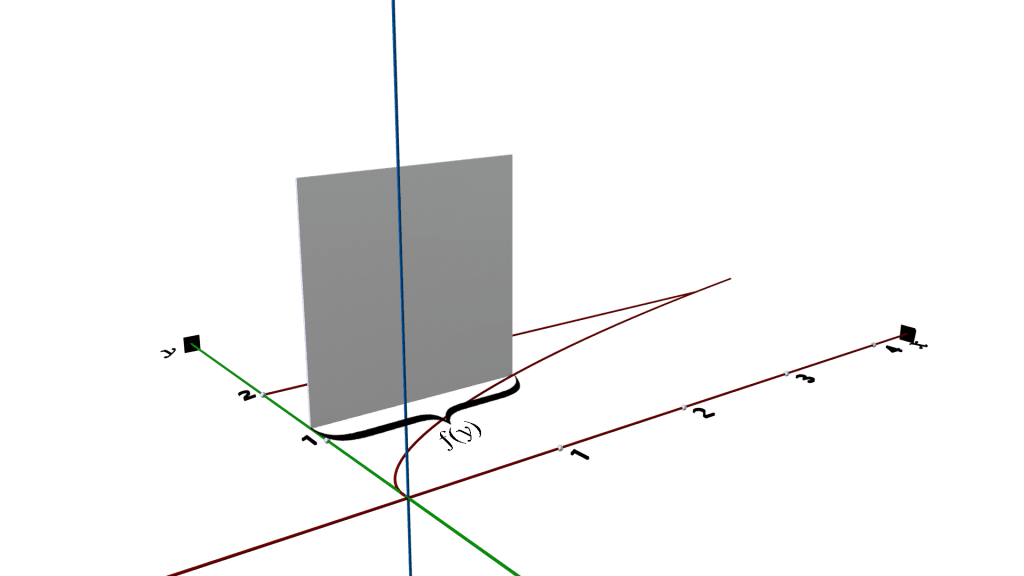
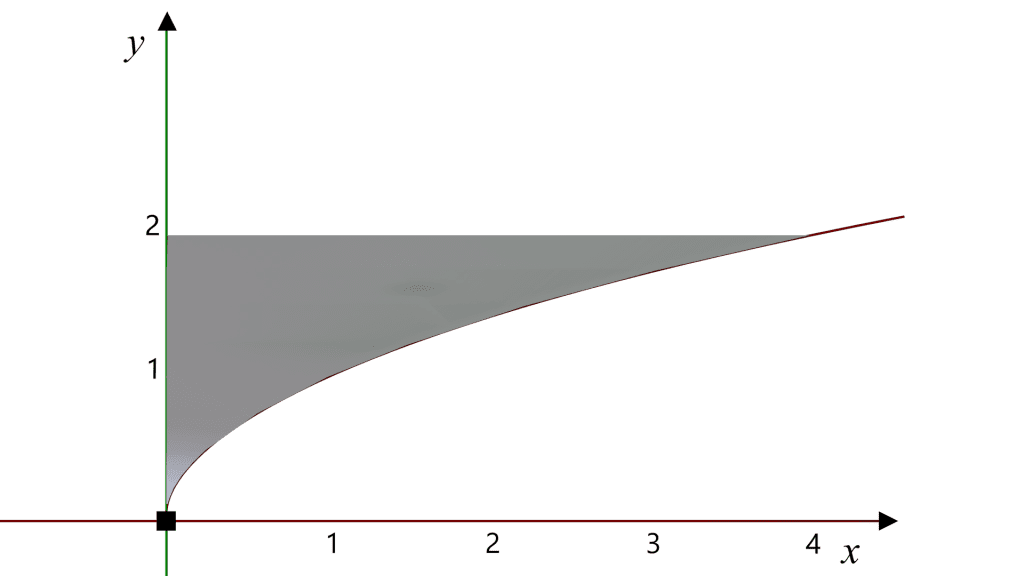
The base of a solid in the region in the first quadrant between the graph of and the x-axis for 0 ≤ x ≤ 4. For the solid, each cross section perpendicular to the y-axis is a square. What is the volume of the solid?
Where S is the side length of the square. We need to rewrite the equation as a function of y (x = f(y)).
The function is given . We need to solve for x. Squaring both sides, we get y2 = x. So we use y2 as our function.
The boundaries are now relative to the y-axis. So c = 0 and d = 2.
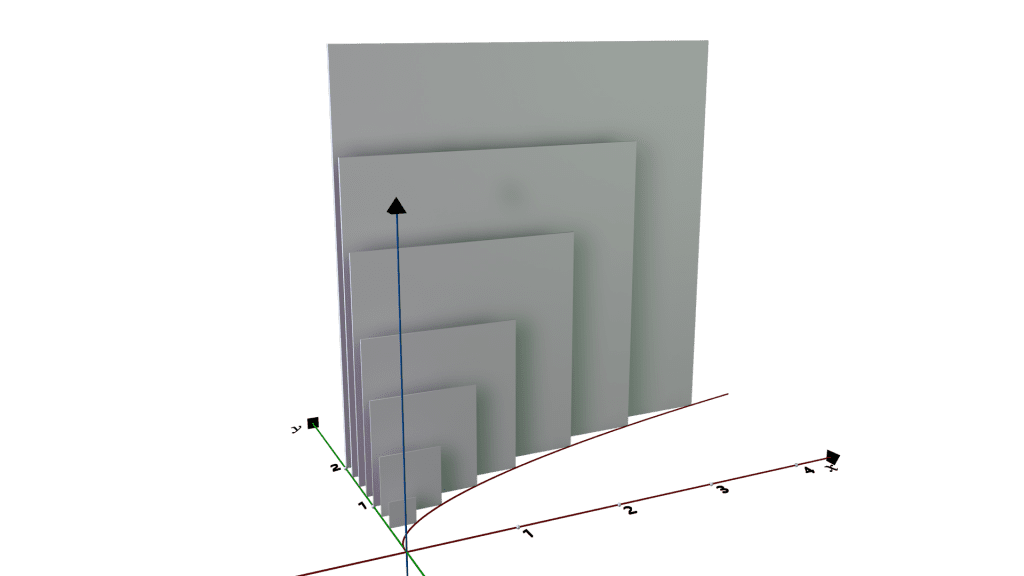
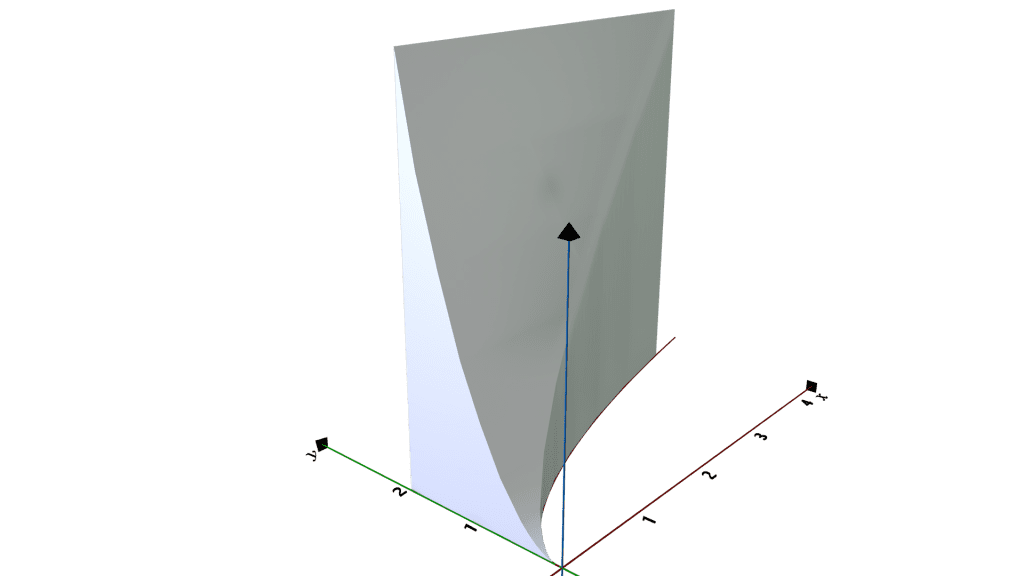

Cross Sections Perpendicular to the y-axis
Application:
This method is often used in calculus to find the volume of solids where slicing perpendicular to an axis results in regular shapes like squares. It’s particularly useful for understanding the volumes of irregular shapes and in various engineering and physics applications.
This approach simplifies complex volume calculations by breaking down the problem into manageable cross sections, integrating to find the sum of their areas over a specified range.
Using the concept of cross sections perpendicular to the y-axis in daily life can help us understand and solve various real-world problems. Here are some practical applications: Cross Sections Perpendicular to the y-axis
Architecture and Construction:
- Building Design: Architects and engineers use cross-sectional analysis to determine the structural integrity and volume of different sections of buildings. For example, when designing a multi-story building, they analyze cross sections to ensure each floor can support the weight above it.
- Bridge Construction: Engineers analyze cross sections of bridges to calculate the amount of materials needed and to ensure the bridge can withstand various loads. Cross Sections Perpendicular to the y-axis
Manufacturing:
- Product Design: In manufacturing, understanding the volume of objects with complex shapes, such as car parts or machinery components, is essential. Engineers use cross-sectional analysis to design molds and ensure parts fit together correctly.
- Quality Control: By analyzing cross sections, manufacturers can check for internal defects or inconsistencies in products, such as air bubbles in castings or uneven thickness in extruded materials.
Medicine:
- Medical Imaging: Techniques like MRI and CT scans produce cross-sectional images of the human body. Doctors use these images to examine organs, detect abnormalities, and plan surgeries with precision.
- Prosthetics Design: When designing custom prosthetics, cross-sectional data helps ensure the prosthetic fits perfectly with the patient’s anatomy.
Environmental Science:
- Topographic Analysis: Environmental scientists use cross-sectional analysis to study landforms and topography. This helps in understanding erosion patterns, planning flood control measures, and assessing the impact of construction projects on the environment.
- Hydrology: In hydrology, cross sections of riverbeds and floodplains are analyzed to predict water flow, manage water resources, and design flood defenses.
Agriculture:
- Irrigation Systems: Farmers use cross-sectional analysis to design efficient irrigation systems. By understanding the topography and soil composition, they can ensure even distribution of water across their fields.
- Soil Analysis: Cross sections of soil samples help in studying soil layers, determining soil health, and planning crop rotation strategies.
Transportation:
- Road and Railway Design: Engineers analyze cross sections of terrain to design roads and railways that are safe and cost-effective. This includes calculating the volume of earth to be moved and ensuring the stability of slopes and embankments.
- Tunnel Construction: Cross-sectional analysis is crucial in tunnel construction to ensure the tunnel can safely pass through different types of rock and soil.







