Loading [MathJax]/extensions/tex2jax.js
About Us Donate

3.4 Ideal Gas Law

Ideal Gas Law

3.4 Gas Laws and Relationships

Pressure and Temperature

When thinking about pressure in the context of gases, remember this key idea: Pressure is the result of gas particles colliding with the walls of their container. The more collisions that occur, the greater the pressure. If you’re asked about pressure on the AP exam, always mention particle collisions with the container walls! Below are the values for standard pressure in various units: Standard Pressure: 1.00 atm = 760 mm Hg = 760 torr = 101.3 kPa
Temperature measures the average kinetic energy of particles in a substance. As temperature increases, the particles move faster. Keep this in mind: higher temperature = faster particle movement. Standard Temperature: 0°C = 273.15 K (°C + 273.15 = K) You’ll see STP (Standard Temperature and Pressure) mentioned often in AP questions. STP conditions are 1 atm and 273.15 K.

Gas Laws & Relationships

Gas laws describe the relationships between pressure, volume, temperature, and moles of gas. These relationships assume the amount of gas is constant.

Boyle’s Law: Pressure-Volume Relationship

Boyle’s Law describes the inverse relationship between pressure and volume at constant temperature. The equation is: P₁V₁ = P₂V₂ When the volume of a gas decreases, particles collide more frequently with the container walls, resulting in increased pressure.

Charles’ Law: Volume-Temperature Relationship

Charles’ Law shows the direct relationship between volume and temperature at constant pressure: V₁/T₁ = V₂/T₂ When the temperature increases, gas particles move faster, causing more collisions and an increase in volume to maintain constant pressure.

Gay-Lussac’s Law: Pressure-Temperature Relationship

Gay-Lussac’s Law describes the direct relationship between pressure and temperature at constant volume: P₁/T₁ = P₂/T₂ As temperature increases, particles collide more often and with greater force, increasing the pressure.

Avogadro’s Law: Volume-Moles Relationship

Avogadro’s Law shows the direct relationship between the volume of a gas and the number of moles (n) of gas: V₁/n₁ = V₂/n₂ Increasing the number of gas particles in a container leads to more collisions, causing the volume to increase to maintain constant pressure.

The Combined Gas Law

The Combined Gas Law combines Boyle’s, Charles’, and Gay-Lussac’s Laws into one equation: P₁V₁/T₁ = P₂V₂/T₂ When solving problems, you can ignore variables that don’t change. For example, if temperature is constant, the equation simplifies to Boyle’s Law: P₁V₁ = P₂V₂.

Gas Law Formula

Gas Law Formula Description
Boyle’s Law P₁V₁ = P₂V₂ At constant T, as pressure increases, volume decreases.
Charles’ Law V₁/T₁ = V₂/T₂ At constant P, as volume increases, temperature increases.
Gay-Lussac’s Law P₁/T₁ = P₂/T₂ At constant V, as pressure increases, temperature increases.
Combined Law P₁V₁/T₁ = P₂V₂/T₂ Combines Boyle’s, Charles’, and Gay-Lussac’s Laws.
Ideal Gas Law PV = nRT Relates pressure, volume, temperature, and moles of gas with the ideal gas constant.

The Ideal Gas Law

The Ideal Gas Law is one of the most important equations you’ll see in AP Chemistry: PV = nRT Where:
  • P = pressure (in atm)
  • V = volume (in L)
  • n = moles of gas
  • R = universal gas constant (0.08206 L·atm/mol·K)
  • T = temperature (in Kelvin)
Remember: Always convert temperature to Kelvin, volume to liters, and pressure to atm when using the ideal gas law! The Ideal Gas Law is almost always on the AP Chemistry exam, so make sure you’re comfortable with it.

Dalton’s Law of Partial Pressure

Dalton’s Law of Partial Pressures states that in a mixture of gases, each gas exerts its own partial pressure, independent of the other gases. The total pressure is the sum of the partial pressures of all the gases: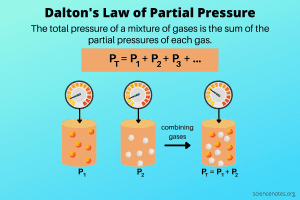 AP Chemistry slyacademy.com P_total = P₁ + P₂ + P₃… To calculate partial pressure, use the mole fraction (X) of a gas: P₁ = X₁ · P_total The mole fraction (X₁) is the ratio of moles of one gas to the total number of moles in the mixture: X₁ = n₁ / (n₁ + n₂ + n₃…) For example, if you have 3 moles of O₂ and 4 moles of H₂ in a container, the mole fraction of O₂ is: X₁ = 3 / (3 + 4) = 3/7 Then, to find the partial pressure of O₂: P₁ = X₁ · P_total

AP Chemistry Reference Table

Be sure to familiarize yourself with the AP Chemistry reference table for this chapter. You’ll be given important constants and formulas during the exam, such as the universal gas constant (R) and the Ideal Gas Law formula.

Gases, Liquids, and Solutions

Gas Laws:
  • Ideal Gas Law: PV=nRTPV = nRT
  • Partial Pressure Formula: PA=Ptotal×XAP_A = P_{total} \times X_A, where XA=moles Atotal molesX_A = \frac{\text{moles A}}{\text{total moles}}
  • Total Pressure: Ptotal=PA+PB+PC+…P_{total} = P_A + P_B + P_C + \ldots 
  • Moles Formula: n=mMn = \frac{m}{M} nn= number of moles, mm= mass, MM= molar mass
  • Temperature Conversion: K=∘C+273K = {}^\circ C + 273 
  • Density Formula: D=mVD = \frac{m}{V} DD= density, mm= mass, VV= volume
  • Kinetic Energy of a Molecule: KEmolecule=12mv2KE_{molecule} = \frac{1}{2} mv^2 
Concentration:
  • Molarity (M): Moles of solute per liter of solution
  • Beer-Lambert Law (Absorbance): A=εbcA = \varepsilon bc  AA= absorbance, ε\varepsilon= molar absorptivity, bb= path length, cc= concentration
Constants & Conversions:
  • Gas Constant (R): 8.314 J mol−1 K−18.314 \, \text{J} \, \text{mol}^{-1} \, \text{K}^{-1}  0.08206 L atm mol−1 K−10.08206 \, \text{L} \, \text{atm} \, \text{mol}^{-1} \, \text{K}^{-1}  62.36 L torr mol−1 K−162.36 \, \text{L} \, \text{torr} \, \text{mol}^{-1} \, \text{K}^{-1} 
  • Pressure Conversions: 1 atm=760 mm Hg=760 torr1 \, \text{atm} = 760 \, \text{mm Hg} = 760 \, \text{torr} 
  • STP (Standard Temperature and Pressure): 273.15 K and 1.0 atm273.15 \, \text{K} \, \text{and} \, 1.0 \, \text{atm} 
  • Molar Volume at STP: Ideal gas at STP = 22.4 L mol −1^{-1} 

Key Practice Question

Let’s practice using Dalton’s Law with the following question: A 10 L container holds a mixture of 3 moles of O₂ and 4 moles of H₂ at a total pressure of 2 atm. What is the partial pressure of O₂?

Step-by-Step Solution:

  1. Find the mole fraction (X₁) of O₂: Mole fraction of O₂ = 3 / (3 + 4) = 3/7
  2. Use Dalton’s Law to find the partial pressure of O₂: P₁ = X₁ · P_total = (3/7) · 2 atm = 0.86 atm

Summary of Key Gas Laws

Law Equation Relationship
Boyle’s Law P₁V₁ = P₂V₂ Pressure and volume (inverse)
Charles’ Law V₁/T₁ = V₂/T₂ Volume and temperature (direct)
Gay-Lussac’s Law P₁/T₁ = P₂/T₂ Pressure and temperature (direct)
Avogadro’s Law V₁/n₁ = V₂/n₂ Volume and moles (direct)
Combined Gas Law P₁V₁/T₁ = P₂V₂/T₂ Pressure, volume, and temperature
Ideal Gas Law PV = nRT Pressure, volume, temperature, moles
Dalton’s Law P_total = P₁ + P₂ + P₃… Total pressure and partial pressures
Mastering these gas laws will help you navigate a wide range of problems on the AP Chemistry exam. Be sure to practice calculating pressures, volumes, and temperatures using these laws, and remember to always include explanations about particle collisions when discussing pressure!

More Sly academy Content