
Table of Contents
ToggleCross Sections Perpendicular to the x-axis (SEMI-CIRCLE)
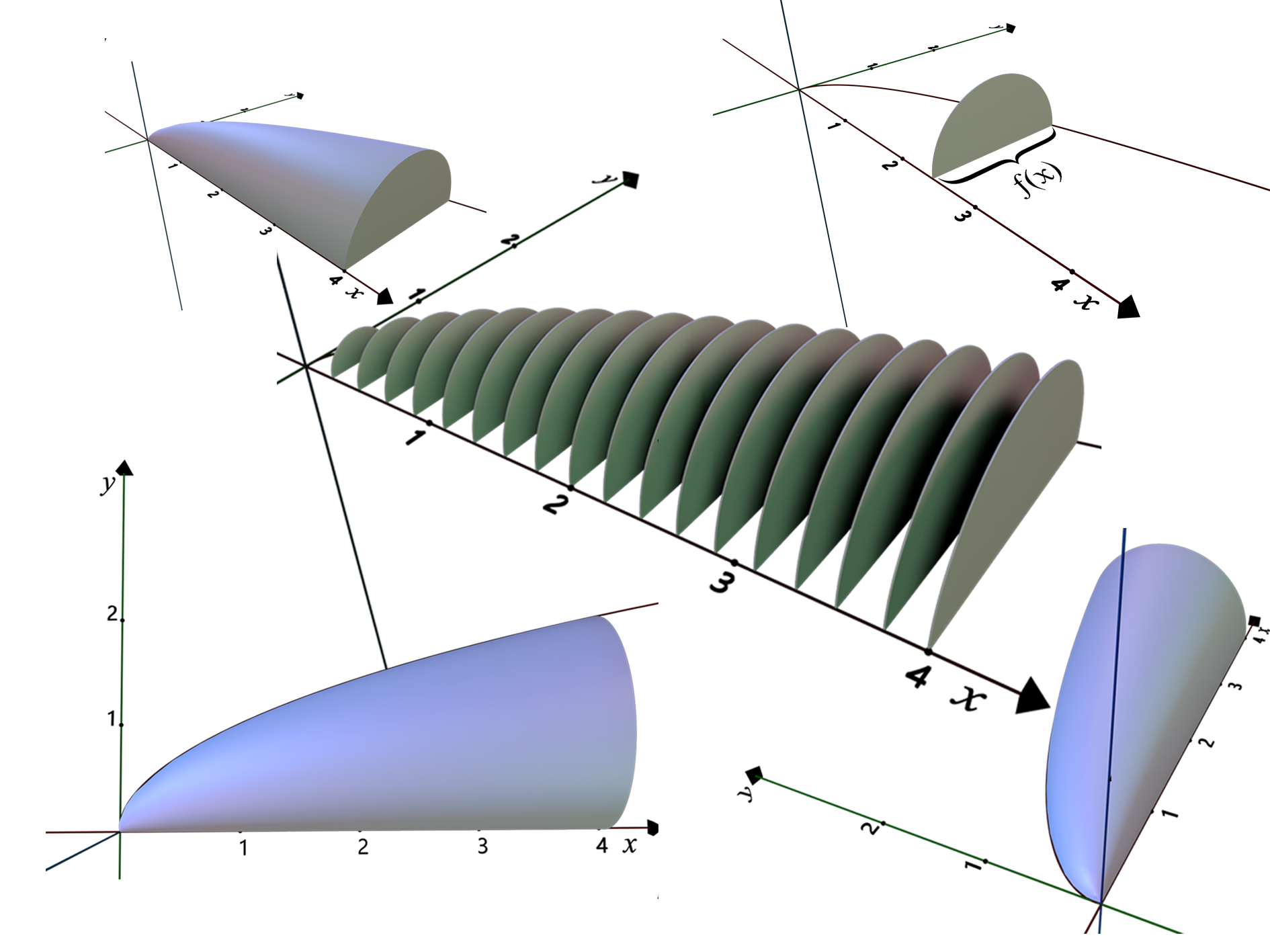
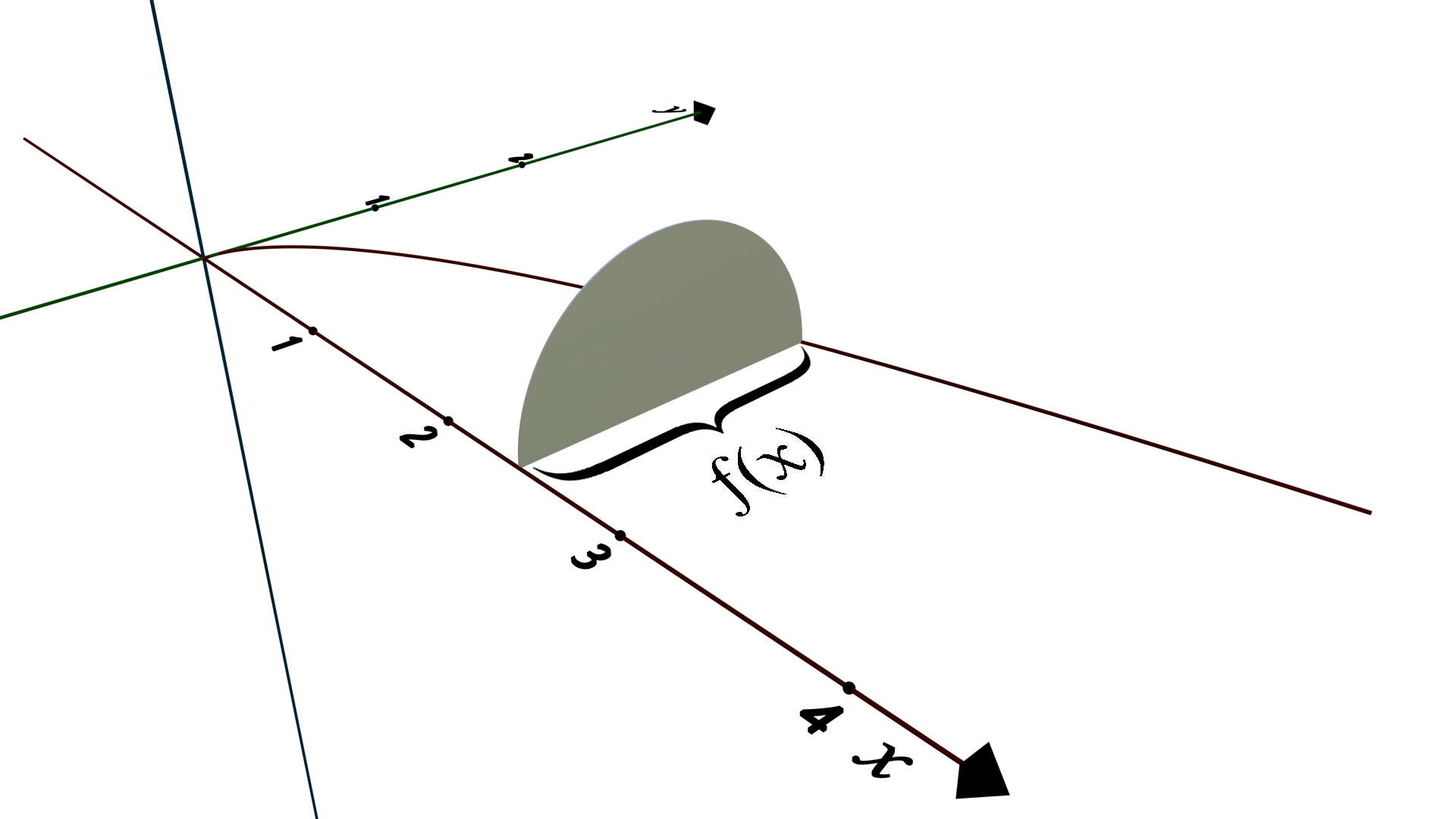
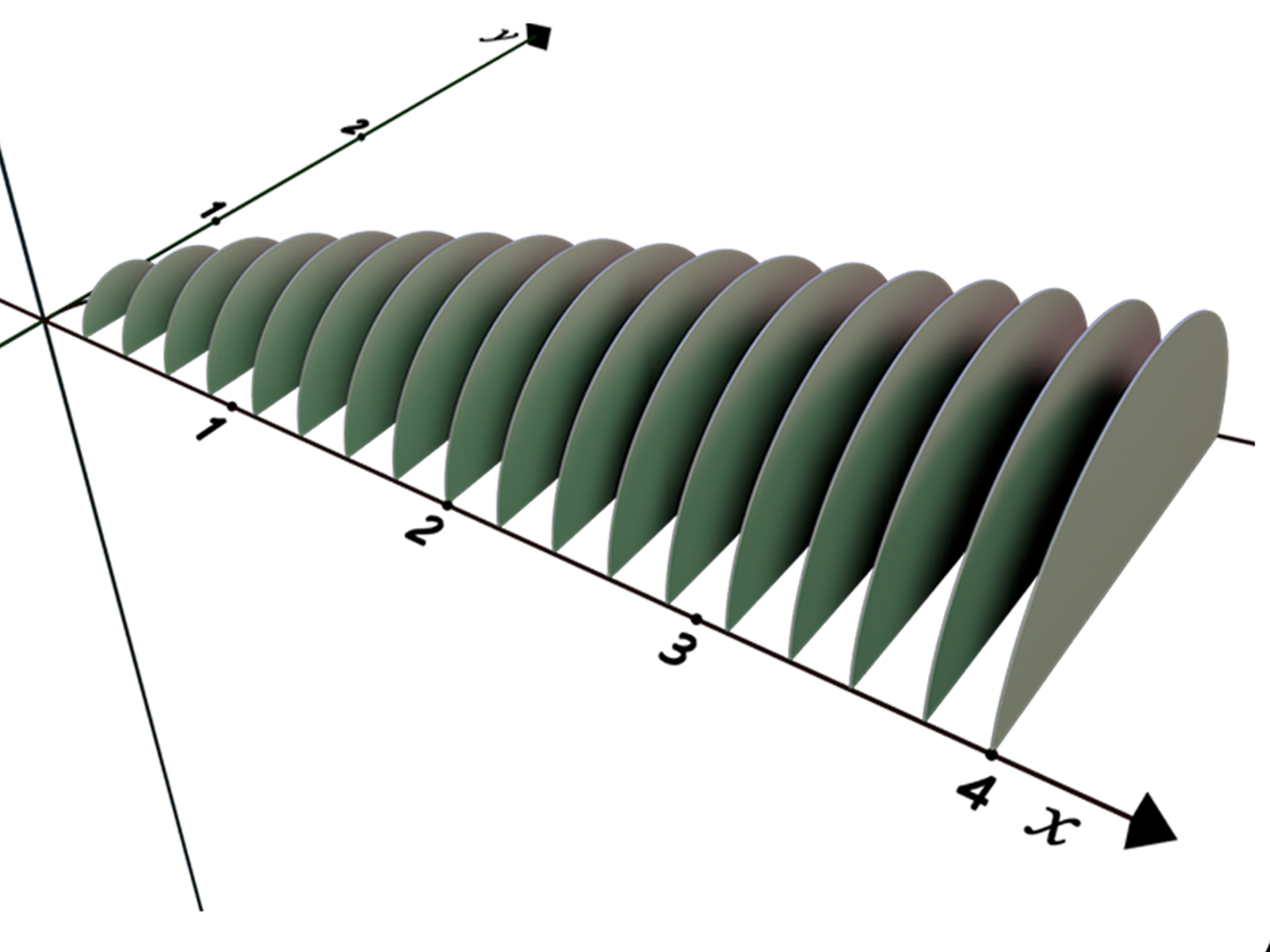
3) The base of a solid in the region in the first quadrant between the graph of and the x-axis for 0 ≤ x ≤ 4. For the solid, each cross section perpendicular to the x-axis is a semi-circle. What is the volume of the solid?

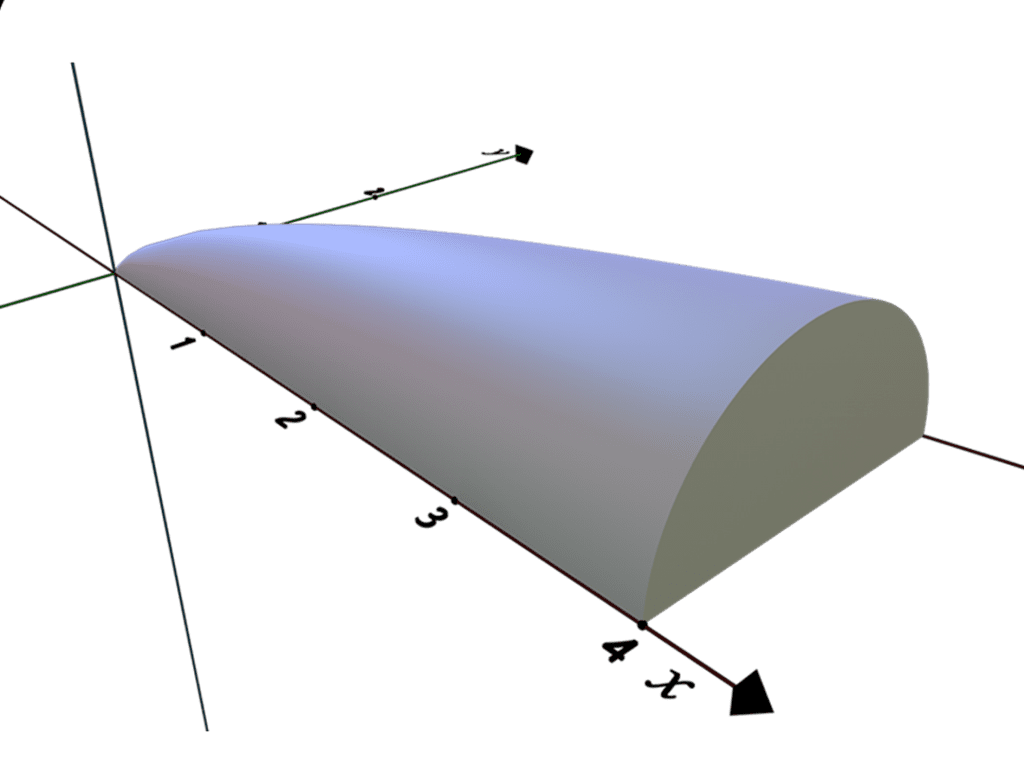

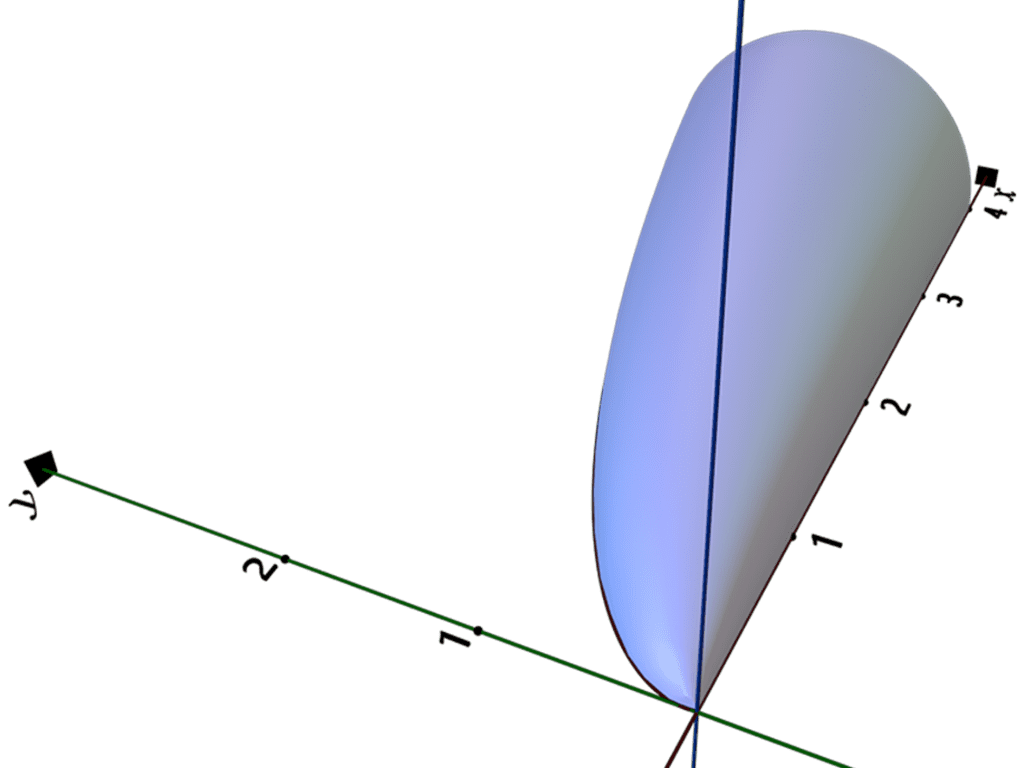

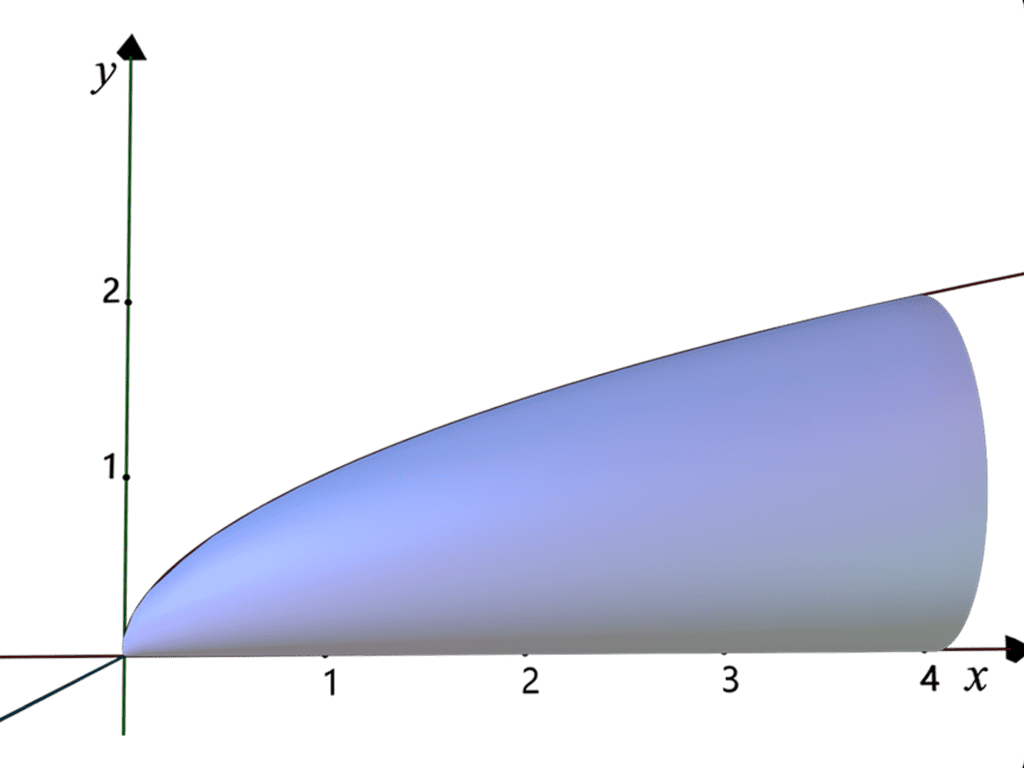

Application of Volumes of Revolution
Volumes of revolution are applied in various fields such as engineering, physics, and medicine to solve practical problems involving the creation of 3D objects from 2D shapes. Here are some applications:
Cross Sections Perpendicular to the x-axis (SEMI-CIRCLE)
1. Engineering
Design and Manufacturing: Engineers often use volumes of revolution to design and manufacture parts with rotational symmetry, such as gears, wheels, and axles. For example, when designing a car wheel, the profile of the wheel can be rotated around the central axis to create the 3D shape of the wheel.
Cross Sections Perpendicular to the x-axis (SEMI-CIRCLE)
Aerospace Engineering: The fuselage of an aircraft can be modeled as a volume of revolution. By rotating the cross-sectional profile of the fuselage around the longitudinal axis, engineers can calculate the volume and surface area, which are critical for determining material requirements and aerodynamic properties.
Cross Sections Perpendicular to the x-axis (SEMI-CIRCLE)
2. Physics
Fluid Dynamics: In fluid dynamics, the shape of a container can affect how fluids behave within it. For example, the shape of a wine glass can be created by revolving a curve around an axis, and the volume of the wine glass can be calculated using the disk or washer method to determine how much liquid it can hold.
Solids of Revolution in Mechanics: Objects such as flywheels or pulleys, which have rotational symmetry, are often analyzed using volumes of revolution to determine their mass distribution and moments of inertia.
Cross Sections Perpendicular to the x-axis (SEMI-CIRCLE)
3. Medicine
Medical Imaging: Volumes of revolution are used in medical imaging techniques such as MRI and CT scans to create 3D models of organs from 2D cross-sectional images. This helps doctors visualize and analyze the structure of organs for diagnostic and surgical planning purposes.
Cross Sections Perpendicular to the x-axis (SEMI-CIRCLE)
Prosthetics and Implants: Designing prosthetic limbs and implants often involves creating objects with rotational symmetry. By using volumes of revolution, designers can calculate the required volume and shape to ensure proper fit and function.
Cross Sections Perpendicular to the x-axis (SEMI-CIRCLE)
Additional Uses of Volumes of Revolution
1. Architecture
- Designing Domes and Arches: Architects use volumes of revolution to design structures like domes and arches. By rotating a curve around an axis, they can create a 3D model of the dome, calculate its volume, and ensure structural integrity. For example, the design of the dome of the Capitol Building in Washington, D.C., can be analyzed using these principles.
Cross Sections Perpendicular to the x-axis (SEMI-CIRCLE)
2. Manufacturing
- Production of Cylindrical Objects: In manufacturing, volumes of revolution are used to design and produce cylindrical objects such as pipes, bottles, and cans. Engineers calculate the volume to determine the amount of material needed and to ensure the efficiency of the manufacturing process. For instance, beverage companies use these calculations to design bottles with the appropriate capacity.
Cross Sections Perpendicular to the x-axis (SEMI-CIRCLE)
3. Astrophysics
- Modeling Celestial Bodies: Astrophysicists use volumes of revolution to model the shapes and volumes of celestial bodies like planets and stars. By rotating a 2D profile around an axis, they can estimate the volume and density of these bodies, which is essential for understanding their physical properties and behavior.
Cross Sections Perpendicular to the x-axis (SEMI-CIRCLE)
4. Pharmacology
- Designing Medical Capsules: In pharmacology, volumes of revolution help design medical capsules and pills. By calculating the volume of the capsule, pharmaceutical companies can determine the correct dosage and ensure the capsule has the appropriate shape and size for ingestion.
Cross Sections Perpendicular to the x-axis (SEMI-CIRCLE)
5. Environmental Science
- Designing Storage Tanks: Environmental scientists use volumes of revolution to design storage tanks for liquids such as water, oil, and chemicals. By revolving a curve around an axis, they can create a model of the tank and calculate its volume, which is crucial for storage and transportation logistics.
6. Automotive Industry
- Designing Fuel Tanks: Automotive engineers use volumes of revolution to design fuel tanks with specific capacities. By revolving a curve around an axis, they can create a 3D model of the tank, ensuring it fits within the vehicle’s design and meets fuel capacity requirements.
7. Cosmetics Industry
- Designing Containers: The cosmetics industry uses volumes of revolution to design containers for products such as lotions, perfumes, and creams. By calculating the volume of these containers, companies can ensure they meet consumer needs and optimize material usage.
Examples:
- Designing a Parabolic Dish: To design a parabolic dish for a satellite, engineers rotate a parabolic curve around its axis to create the dish’s 3D shape, allowing them to calculate its volume and surface area for efficient signal reception.
- Modeling a Torus: In topology, mathematicians use volumes of revolution to model a torus (a doughnut-shaped object) by rotating a circle around an axis outside the circle. This is useful in both theoretical mathematics and practical applications like designing inflatable tubes.

Graphs of Inverse Trigonometry Functions
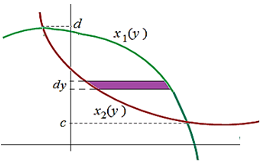

AREAS btw CURVES and VOLUMES of REVOLUTIONS


Word to JPEG – Convert Word files to JPEG Images Online


WebP to JPEG – Convert WebP to JPEG


HEIC to JPG – Convert HEIC Images to JPEG Format